From Mechanics

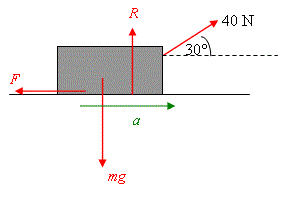
We have drawn all the forces acting on the block in the figure. The acceleration is represented by the vector \displaystyle a.

Applying Newton´s Second Law \displaystyle F=ma horisontally,

\displaystyle \begin{align} & \to :\ 40\cos {{30}^{\circ }}-F=ma \\ \end{align} ration is represented by the vector \displaystyle a.

Note that \displaystyle R is NOT equal to \displaystyle mg here as the string has a component force in the vertical direction! In fact one has,

\displaystyle \begin{align} & \uparrow :\ R+40\sin {{30}^{\circ }}-mg=0 \\ & \\ & F=mg-40\sin {{30}^{\circ }}=3\times 9\textrm{.}81-40\times \frac{1}{2}=29\textrm{.}43-20=9\textrm{.}43\ \text{N} \\ \end{align}

Using the friction equation gives

\displaystyle \begin{align} & F=\mu R \\ & \\ & F =0\textrm{.}5 \times 9\textrm{.}43 = 4\textrm{.}72 \ \text{N} \\ \end{align}