Solution to Test Paper 2
From Mechanics
| 1 (a) | \displaystyle \begin{align}
& 44\textrm{.}1=\frac{1}{2}\times 9\textrm{.}8{{t}^{2}} \\ & t=\sqrt{\frac{44\textrm{.}1}{4\textrm{.}9}}=3\text{ s} \\ & \\ & \text{OR} \\ & \\ & s=\frac{1}{2}\times 9\textrm{.}8\times {{3}^{2}}=44\textrm{.}1 \\ & \therefore \text{Hits ground after 3 seconds} \\ \end{align} AG
| M1
A1 A1
(A1) (A1)
| (3 marks) | M1: Use of constant acceleration
equation with \displaystyle v=0 A1: Correct equation A1: Correct \displaystyle s
|
| 1 (b) | \displaystyle \begin{align}
& {{v}^{2}}={{0}^{2}}+2\times 9\textrm{.}8\times 44\textrm{.}1 \\ & v=\sqrt{864\textrm{.}36}=29\textrm{.}4m \\ & \\ & \text{OR} \\ & \\ & v=0+9\textrm{.}8\times 3 \\ & v=29\textrm{.}4 \\ \end{align}
| M1
A1 A1
| (3 marks)
| M1: Use of constant acceleration equation with \displaystyle v=0
A1: Correct equation. A1: Correct \displaystyle v.
|
| 1 (c) | Air resistance would slow the ball down. | B1 | (1 mark) | B1: Sensible statement about air resistance. |
| (7 marks) | ||||
| 2 (a) | 
| B1 | (1 mark) | B1: Correct horizontal forces.
Ignore any vertical forces.
|
| 2 (b) | \displaystyle P = 900 \text{N} | B1 | (1 mark) | B1: Correct value for \displaystyle P. |
| 2 (c) | \displaystyle \begin{align}
& P-900=2000\times 1\textrm{.}2 \\ & P=2400+900=3300 \text{N} \\ \end{align} | M1
A1 A1 | (1 mark) | M1: Three term equation of motion
A1: Correct equation A1: Correct \displaystyle P.
|
| 2 (d) | \displaystyle \begin{align}
& 800-900=2000a \\ & a=\frac{-100}{2000}=-0\textrm{.}05 \text{ m}{{\text{s}}^{-2}} \\ & \text{Car is slowing down} \\ \end{align}
| M1
A1 A1 A1 | (4 marks) | M1: Three term equation of motion
A1: Correct equation A1: Correct \displaystyle a A1: Correct statement
|
| (9 marks) | ||||
| 3 (a) | \displaystyle R=20\times 9\textrm{.}8=196 N | M1
A1 | (2 marks) | M1: Use of \displaystyle R=mg
A1: Correct \displaystyle R.
|
| 3 (b) | \displaystyle F=0\textrm{.}4\times 196=78\textrm{.}4 N | M1
A1 | (2 Marks) | M1: Use of \displaystyle F=\mu R
A1: Correct \displaystyle F.
|
| 3 (c) | \displaystyle \begin{align}
& 100-78 \textrm{.}4=20a \\ & a=\frac{100-78 \textrm{.}4}{20}=1 \textrm{.}08 \text{ m}{{\text{s}}^{-2}} \\ \end{align} | M1
A1 A1 | (3 marks) | M1: Three term equation of motion
A1: Correct equation A1: Correct \displaystyle a.
|
| (8 marks) | ||||
| 4 (a) | 
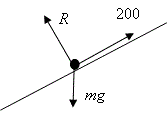
| B1 | (1 mark) | B1: Correct force diagram |
| 4 (b) | \displaystyle \begin{align}
& 100a=200-980\sin 5{}^\circ \\ & a=\frac{200-980\sin 5{}^\circ }{100}=1\textrm{.}15\ \text{m}{{\text{s}}^{-2}} \\ \end{align} | M1A1
M1 A1 | (4 marks) | M1: Three term equation of motion
A1: Correct equation M1: Rearranging equation. A1: Correct \displaystyle a.
|
| 4 (c) |
\displaystyle \begin{align} & s=0\times 5+\frac{1}{2}\times 1\textrm{.}15\times {{5}^{2}} \\ & =14\textrm{.}4\ \text{m} \\ \end{align} | M1
A1 A1 | (3 marks) | M1: Using a constant acceleration equation
A1: Correct equation A1: Correct distance.
|
| (8 marks) | ||||
| 5 (a) | \displaystyle \mathbf{v}=(6\mathbf{i}+4\mathbf{j})+(0\textrm{.}2\mathbf{i}-0\textrm{.}4\mathbf{j})t | M1
A1 | (2 marks) | M1: Use of \displaystyle \mathbf{v}=\mathbf{u}+\mathbf{a}t
A1: Correct expression
|
| 5 (b) | \displaystyle \begin{align}
& 4-0\textrm{.}4t=0 \\ & t=10 \ \text{s}\\ \end{align} | M1
A1 A1 | (3 marks) | M1: Using \displaystyle \mathbf{j} component equal to zero.
A1: Correct equation. A1: Correct time. |
| 5 (c) | \displaystyle \begin{align}
& \mathbf{r}=(6\mathbf{i}+4\mathbf{j})\times 30+\frac{1}{2}(0\textrm{.}2\mathbf{i}-0\textrm{.}4\mathbf{j})\times {{30}^{2}} \\ & =270\mathbf{i}-60\mathbf{j} \\ & r=\sqrt{{{270}^{2}}+{{60}^{2}}}=277\ \text{m} \\ \end{align} | M1
A1 M1 A1 | (4 marks) | M1: Using \displaystyle \mathbf{r}=\mathbf{u}t+\frac{1}{2}\mathbf{a}{{t}^{2}}
A1: Correct position vector. M1: Finding distance. A1: Correct distance |
| (8 marks)
|
KEY M1: Method Mark
A1: Accuracy Mark following a method mark
B1: Accuracy Mark not following a method mark
AG: Answer Given in Question – Working must justify answer.
TOTAL: 40 Marks
