From Mechanics
Solutions
| 1 (a)
| \displaystyle \begin{align}
& {{0}^{2}}={{7}^{2}}+2(-9\textrm{.}8)s \\
& s=\frac{49}{19\textrm{.}6}=2\textrm{.}5 \\
& \text{Max Height }=\text{ 5}+\text{2}\text{.5}=\text{7}\text{.5 m} \\
\end{align}
| (3 marks)
|
| 1 (b)
| \displaystyle \begin{align}
& 0=7-9\textrm{.}8t \\
& t=\frac{7}{9\textrm{.}8}=0\textrm{.}714\text{ s} \\
\end{align}
| (3 marks)
|
|
|
| (6 marks)
|
| 2 (a)
| \displaystyle \begin{align}
& T-800\times 9\textrm{.}8=800\times 0\textrm{.}2 \\
& T=\text{7840}+\text{160}=\text{8000 N} \\
\end{align}
| (3 marks)
|
| 2 (b)
| \displaystyle \begin{align}
& T-800\times 9\textrm{.}8=800\times (-0\textrm{.}2) \\
& T=\text{7840}-\text{160}=\text{7680 N} \\
\end{align}
| (3 marks)
|
| 2 (c)
| \displaystyle T=800\times 9\textrm{.}8=7840\text{ N}
| (1 mark)
|
|
|
| (7 marks)
|
| 3 (a)
| 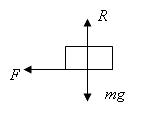
| (1 mark)
|
| 3 (b)
| \displaystyle F=2\times 1\textrm{.}8=3\textrm{.}6\text{ N}
| (2 marks)
|
| 3 (c)
| \displaystyle R=2\times 9\textrm{.}8=19\textrm{.}6\text{ N}
| (2 marks)
|
| 3 (d)
| \displaystyle \begin{align}
& 3 \textrm{.}6=19 \textrm{.}6\mu \\
& \mu =\frac{3 \textrm{.}6}{19 \textrm{.}6}=0 \textrm{.}184 \\
\end{align}
| (3 marks)
|
|
|
| (8 marks)
|
| 4 (a)
| 
| (1 mark)
|
| 4 (b)
| \displaystyle \begin{align}
& R+T\sin 30{}^\circ =200\times 9 \textrm{.}8 \\
& R+0 \textrm{.}5T=1960 \\
& R=1960-0 \textrm{.}5T \\
\end{align}
AG
| (3 marks)
|
| 4 (c)
| \displaystyle
\displaystyle \begin{align}
& F=T\cos 30{}^\circ \\
& T\cos 30{}^\circ =0\textrm{.}6(1960-0\textrm{.}5T) \\
& T(\cos 30{}^\circ +0\textrm{.}3)=1176 \\
& T=\frac{1176}{(\cos 30{}^\circ +0\textrm{.}3)}=1010\text{ N} \\
\end{align}
| (4 marks)
|
|
|
| (8 marks)
|
| 5 (a)
| \displaystyle \begin{align}
& 5\mathbf{i}-2\mathbf{j}=4\mathbf{i}+3\mathbf{j}+10\mathbf{a} \\
& \mathbf{a}=\frac{1}{10}(\mathbf{i}-5\mathbf{j})=\left( 0\textrm{.}1\mathbf{i}-0\textrm{.}5\mathbf{j} \right)\text{ m}{{\text{s}}^{\text{-2}}} \\
\end{align}
| (3 marks)
|
| 5 (b)
| \displaystyle \mathbf{r}=(4\mathbf{i}+3\mathbf{j})t+0\textrm{.}5(0\textrm{.}1\mathbf{i}-0\textrm{.}5\mathbf{j}){{t}^{2}}
| (2 marks)
|
| 5 (c)
| \displaystyle \begin{align}
& \mathbf{r}=(4t+0\textrm{.}05{{t}^{2}})\mathbf{i}+(3t-0\textrm{.}25{{t}^{2}})\mathbf{j} \\
& 3t-0\textrm{.}25{{t}^{2}}=0 \\
& t(3-0\textrm{.}25t)=0 \\
& t=0\text{ or }t=\frac{3}{0\textrm{.}25}=12\text{ s} \\
& t=12\text{ s} \\
\end{align}
| (3 marks)
|
| 5 (d)
| \displaystyle \begin{align}
& \mathbf{v}=(4+0\textrm{.}1t)\mathbf{i}+(3-0\textrm{.}5t)\mathbf{j} \\
& 3-0\textrm{.}5t=0 \\
& t=6 \text{ s} \\
\end{align}
| (3 marks)
|
|
|
| (11 marks)
|
AG: Answer Given in Question – Working must justify answer.