Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
No jsMath TeX fonts found -- using image fonts instead.
These may be slow and might not print well.
Use the jsMath control panel to get additional information.
jsMath Control PanelHide this Message

jsMath

Introduction to forces

From Mechanics

(Difference between revisions)
Jump to: navigation, search
Line 17: Line 17:
<math>G=6.67\times 10^{-11}\text{ kg}^{\text{-1}}\text{m}^{\text{3}}\text{s}^{\text{-2}}</math>
<math>G=6.67\times 10^{-11}\text{ kg}^{\text{-1}}\text{m}^{\text{3}}\text{s}^{\text{-2}}</math>
 +
 +
[[Image:Gravitation.gif]]
'''Gravity on Earth'''
'''Gravity on Earth'''
The force of gravity is often called the weight.
The force of gravity is often called the weight.
 +
Line 27: Line 30:
& g=9.8\text{ ms}^{\text{-2}} \\
& g=9.8\text{ ms}^{\text{-2}} \\
\end{align}</math>
\end{align}</math>
 +
Line 84: Line 88:
<math>R_{1}+R_{2}+R_{3}+R_{4}=215600</math>
<math>R_{1}+R_{2}+R_{3}+R_{4}=215600</math>
 +
 +
 +
 +
'''[[Example 2.3]]'''
 +
 +
A box of mass 30 kg is at rest on a table.
 +
 +
(a) Calculate the weight of the box.
 +
 +
(b) State the magnitude of the upward force that the table exerts on the box.
 +
 +
'''Solution'''
 +
 +
(a)
 +
 +
<math>\begin{align}
 +
& W=30\times 9.8 \\
 +
& =294\text{ N}
 +
\end{align}</math>
 +
 +
(b)
 +
An upward force of 294 N must act for the box to remain in equilibrium.
 +
 +
 +
 +
'''[[Example 2.4]]'''
 +
 +
A satellite, of mass 400 kg, is at a height of 12 km above the surface of the earth. Find the magnitude of the gravitational attraction on the satellite.
 +
Data:
 +
<math>G=6.67\times {{10}^{-11}}</math>
 +
kg-1m3s-2
 +
Radius of earth
 +
 +
<math>=6.37\times {{10}^{6}}</math>
 +
m
 +
Mass of earth
 +
 +
<math>=5.98\times {{10}^{24}}</math>
 +
kg
 +
 +
'''Solution'''
 +
 +
 +
<math>\begin{align}
 +
& \frac{G{{m}_{1}}{{m}_{2}}}{{{d}^{2}}}=\frac{6.67\times {{10}^{-11}}\times 400\times 5.98\times {{10}^{24}}}{{{\left( 6.37\times {{10}^{6}}+12000 \right)}^{2}}} \\
 +
& =3917\text{ N}
 +
\end{align}</math>
 +
 +
 +
'''
 +
[[Example 2.5]]'''
 +
 +
 +
The sun has mass
 +
<math>1.99\times {{10}^{30}}</math>
 +
kg. The mean distance of the earth from the sun is approximately
 +
<math>1.5\times {{10}^{11}}</math>
 +
m.
 +
 +
(a) Calculate the force that the sun exerts on the earth.
 +
(b) State the force that the earth exerts on the sun.
 +
(c) Explain why this force varies.
 +
 +
'''Solution'''
 +
 +
(a)
 +
 +
<math>\begin{align}
 +
& \frac{G{{m}_{1}}{{m}_{2}}}{{{d}^{2}}}=\frac{6.67\times {{10}^{-11}}\times 1.99\times {{10}^{30}}\times 5.98\times {{10}^{24}}}{{{\left( 1.5\times {{10}^{11}} \right)}^{2}}} \\
 +
& =3.53\times {{10}^{22}}\text{ N}
 +
\end{align}</math>
 +
 +
(b)
 +
3.53 × 1022 N
 +
 +
(c) The distance between the earth and the sun varies.

Revision as of 10:28, 16 March 2009

Newton's First Law

A particle will move with a constant velocity or remain at rest if the resultant force on the particle is zero.

Equilibrium

If the resultant force on a particle is zero, then the forces acting on the particle are said to be in equilibrium.

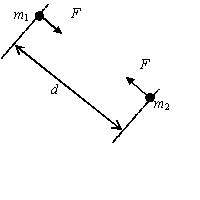
The Universal Law of Gravitation


F=d2Gm1m2


G=66710−11 kg-1m3s-2


Image:Gravitation.gif

Gravity on Earth

The force of gravity is often called the weight.


F=mgg=98 ms-2


Data


Radius of Earth is 637106 metres

Mass of Earth is 5981024 kg


Example 2.1

Describe whether or not the forces acting on the following objects are in equilibrium:

(a) A passenger in a train that travels at a constant speed.

(b) A hot air balloon rising at a constant rate.

(c) A stone dropped into a very deep well full of water.

Solution

(a) Yes, if it is travelling in a straight line.

(b) Yes, if it is travelling in a straight line.

(c) Yes, if it reaches a terminal velocity, so that it is travelling in a straight line at a constant speed.


Example 2.2

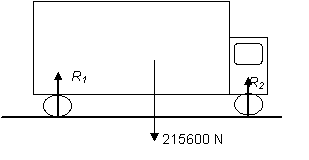
Find the magnitude of the force of gravity (weight) acting on a lorry of mass 22 tonnes.

Solution

This is calculated using the fact that the weight is given by mg.


mg=2200098=215600 N


The diagram shows the lorry and its weight.


Note that reaction forces also act upwards on each wheel.


R1+R2+R3+R4=215600


Example 2.3

A box of mass 30 kg is at rest on a table.

(a) Calculate the weight of the box.

(b) State the magnitude of the upward force that the table exerts on the box.

Solution

(a)

W=3098=294 N

(b) An upward force of 294 N must act for the box to remain in equilibrium.


Example 2.4

A satellite, of mass 400 kg, is at a height of 12 km above the surface of the earth. Find the magnitude of the gravitational attraction on the satellite. Data: G=66710−11 kg-1m3s-2 Radius of earth

=637106 m Mass of earth

=5981024 kg

Solution


d2Gm1m2=637106+12000266710−114005981024=3917 N


Example 2.5


The sun has mass 1991030 kg. The mean distance of the earth from the sun is approximately 151011 m.

(a) Calculate the force that the sun exerts on the earth. (b) State the force that the earth exerts on the sun. (c) Explain why this force varies.

Solution

(a)

d2Gm1m2=151011266710−1119910305981024=3531022 N

(b) 3.53 × 1022 N

(c) The distance between the earth and the sun varies.

Retrieved from "http://wiki.sommarmatte.se/wikis/2009/bridgecoursemechanics/index.php/Introduction_to_forces"