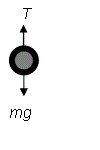Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
No jsMath TeX fonts found -- using image fonts instead.
These may be slow and might not print well.
Use the jsMath control panel to get additional information.
jsMath Control PanelHide this Message

jsMath

Solution 3.3

From Mechanics

(Difference between revisions)
Jump to: navigation, search
(New page: Image:2.1.gif First consider the lower mass. If <math>T=T1</math> is the tension in the lower string then <math>mg=7\times 9\textrm{.}8=68\textrm{....)
Current revision (17:08, 26 March 2011) (edit) (undo)
 
Line 4: Line 4:
First consider the lower mass.
First consider the lower mass.
-
If <math>T=T1</math> is the tension in the lower string then
+
If <math>T={{T}_{1}}</math> is the tension in the lower string then
Line 11: Line 11:
giving
giving
-
<math>T1=68\textrm{.}6\ \text{N}</math>
+
<math>{{T}_{1}}=68\textrm{.}6\ \text{N}</math>
-
If <math>T=T2</math> is the tension in the upper string then the two masses are regarded as one particle with total mass 15 kg.
+
If <math>T={{T}_{2}}</math> is the tension in the upper string then the two masses are regarded as one particle with total mass 15 kg.
Thus in this case
Thus in this case
Line 23: Line 23:
giving
giving
-
<math>T2=147\ \text{N}</math>
+
<math>{{T}_{2}}=147\ \text{N}</math>

Current revision

Image:2.1.gif

First consider the lower mass.

If T=T1 is the tension in the lower string then


mg=79.8=68.6 N


giving T1=68.6 N


If T=T2 is the tension in the upper string then the two masses are regarded as one particle with total mass 15 kg.

Thus in this case


mg=159.8=147 N


giving T2=147 N

Retrieved from "http://wiki.sommarmatte.se/wikis/2009/bridgecoursemechanics/index.php/Solution_3.3"