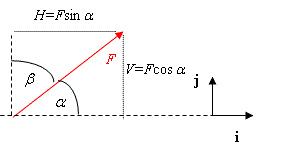Solution 4.5d
From Mechanics
(Difference between revisions)
(New page: Image:teori4.gif Using <math>{{F}^{2}}={{H}^{2}}+{{V}^{2}}</math> and <math>\tan \alpha =\frac{V}{H} </math> Here <math>V=-7\ \text{N}</math> and <math>H=-5\ \text{N}</math>...) |
|||
| Line 21: | Line 21: | ||
| - | <math>F=8.6\ \text{N}</math> | + | <math>F=8\textrm{.}6\ \text{N}</math> |
Current revision
Using \displaystyle {{F}^{2}}={{H}^{2}}+{{V}^{2}}
and
\displaystyle \tan \alpha =\frac{V}{H}
Here
\displaystyle V=-7\ \text{N} and \displaystyle H=-5\ \text{N}.
Substituting the given values
\displaystyle {{F}^{2}}={{\left( -5 \right)}^{2}}+{{\left( \grave{\ }-7 \right)}^{2}}=74
giving
\displaystyle F=8\textrm{.}6\ \text{N}
Also
\displaystyle \tan \alpha =\frac{-7}{-5}=1\textrm{.}4 giving
\displaystyle \alpha =-125\textrm{.}5{}^\circ
Here the solution in the third quadrant has been chosen.