From Mechanics

Revision as of 14:03, 2 September 2010 (edit) Ian (Talk | contribs) ← Previous diff		Current revision (17:03, 10 March 2011) (edit) (undo) Ian (Talk | contribs)
Line 30:		Line 30:
	<math>\begin{align}		<math>\begin{align}
	& {{R}_{A}}+114\textrm{.}3=49+196 \\		& {{R}_{A}}+114\textrm{.}3=49+196 \\
-	& {{R}_{A}}=49+196-114\textrm{.}3=130.7\text{ N} \\	+	& {{R}_{A}}=49+196-114\textrm{.}3=130\textrm{.}7\text{ N} \\
	\end{align}</math>		\end{align}</math>

---

Current revision

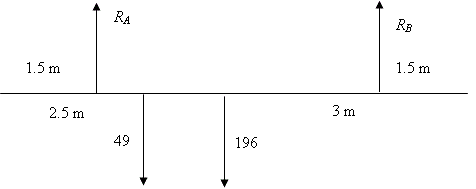
The diagram shows the forces acting.

Taking moments about the left hand support:

\displaystyle \begin{align} & {{R}_{B}}\times 3=49\times 1+196\times 1\textrm{.}5 \\ & {{R}_{B}}=\frac{49\times 1+196\times 1\textrm{.}5}{3}=114\textrm{.}3\text{ N} \\ \end{align}

Taking moments about the right hand support:

\displaystyle \begin{align} & {{R}_{A}}\times 3=49\times 2+196\times 1\textrm{.}5 \\ & {{R}_{B}}=\frac{49\times 2+196\times 1\textrm{.}5}{3}=130\textrm{.}7\text{ N} \\ \end{align}

Or:

\displaystyle \begin{align} & {{R}_{A}}+114\textrm{.}3=49+196 \\ & {{R}_{A}}=49+196-114\textrm{.}3=130\textrm{.}7\text{ N} \\ \end{align}