Solution 10.1c
From Mechanics
(Difference between revisions)
(New page: Image:10.1.gif Here <math>T</math> is the tension in the cable and <math>a</math> is the acceleration of the packet. The acceleration is in the opposite direction to the motion.This ...) |
|||
| Line 3: | Line 3: | ||
Here <math>T</math> is the tension in the cable and <math>a</math> is the acceleration of the packet. | Here <math>T</math> is the tension in the cable and <math>a</math> is the acceleration of the packet. | ||
| - | The acceleration is in the opposite direction to the motion.This means mathematically the acceleration is negative | + | The acceleration is in the opposite direction to the motion.This means mathematically the acceleration is negative, <math>a=-0 \textrm{.}1\text{ m}{{\text{s}}^{\text{-2}}}</math> |
Applying Newton's second law | Applying Newton's second law | ||
Current revision
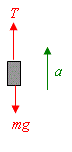
Here \displaystyle T is the tension in the cable and \displaystyle a is the acceleration of the packet.
The acceleration is in the opposite direction to the motion.This means mathematically the acceleration is negative, \displaystyle a=-0 \textrm{.}1\text{ m}{{\text{s}}^{\text{-2}}}
Applying Newton's second law
\displaystyle \begin{align} & \uparrow :\ \text{Resultant}\ \text{Force}=T-300\times 9 \textrm{.}81= ma = 300\times (-0 \textrm{.}1) \\ & \\ & T=-30+2943\approx 2910\ \text{N} \\ \end{align}