Testsida3

Förberedande kurs i matematik

(Skillnad mellan versioner)
Hoppa till: navigering, sök
Rad 2: Rad 2:
<div class="ovning">
<div class="ovning">
{| width="100%" cellspacing="10px"
{| width="100%" cellspacing="10px"
-
Hitta alla extrempunkter på intervallet <math>(-2,2)</math>.
+
Hitta antalet lokala maximi- och minimipunkter på intervallet <math>(-2,2)</math> först grafiskt och sedan genom att hitta derivatans nollställen.
|a)
|a)
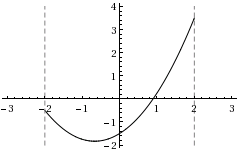
|[[Bild:Kap4plotA.png|left]]<math>f(x)=\frac{3x^2}{4} +x-3/2</math>
|[[Bild:Kap4plotA.png|left]]<math>f(x)=\frac{3x^2}{4} +x-3/2</math>
Rad 13: Rad 13:
|-
|-
|d)
|d)
-
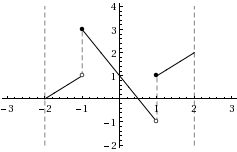
|[[Bild:Kap4plotD.png|left]]<math>f(x)=\begin{cases}-2x+4&\text{om }x<-1\\-2x+4&\text{om }x<-1\\-2x+4&\text{om }x<-1\end{cases}</math>
+
|[[Bild:Kap4plotD.png|left]]<math>f(x)=\begin{cases}-2x+4&\text{om }x<-1\\2&\text{om }-1\leq x\leq 1\\2x+4&\text{om }x>1\end{cases}</math>
|-
|-
|e)
|e)
Rad 19: Rad 19:
|-
|-
|f)
|f)
-
|[[Bild:Kap4plotF.png|left]]<math>f(x)=\frac{3x^2}{4} +x-3/2</math>
+
|[[Bild:Kap4plotF.png|left]]<math>f(x)=\begin{cases}-2x-\frac{1}{2}&\text{om }x<\frac{1}{2}\\x&\text{om }x\geq\frac{1}{2}\end{cases}</math>
||
||
|}
|}
</div>{{#NAVCONTENT:Svar| Svar 4.2.2 | Lösning 4.2.2 a | Lösning 4.4.2.a }}
</div>{{#NAVCONTENT:Svar| Svar 4.2.2 | Lösning 4.2.2 a | Lösning 4.4.2.a }}

Versionen från 19 juni 2012 kl. 14.21

Övning 4.2.2

Hitta antalet lokala maximi- och minimipunkter på intervallet \displaystyle (-2,2) först grafiskt och sedan genom att hitta derivatans nollställen.
a)
\displaystyle f(x)=\frac{3x^2}{4} +x-3/2
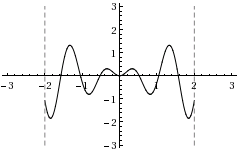
b)
\displaystyle f(x)=x\sin{(6x)}
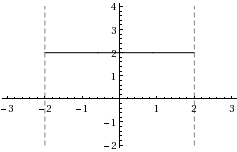
c)
\displaystyle f(x)=2
d)
\displaystyle f(x)=\begin{cases}-2x+4&\text{om }x<-1\\2&\text{om }-1\leq x\leq 1\\2x+4&\text{om }x>1\end{cases}
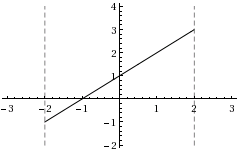
e)
\displaystyle f(x)=x+1
f)
\displaystyle f(x)=\begin{cases}-2x-\frac{1}{2}&\text{om }x<\frac{1}{2}\\x&\text{om }x\geq\frac{1}{2}\end{cases}
Svar
Svar 4.2.2
Lösning 4.2.2 a
Lösning 4.4.2.a
Den här artikeln är hämtad från http://wiki.sommarmatte.se/wikis/forberedandematte/index.php/Testsida3