Lösung 3.3:1b

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel))
Zeile 5: Zeile 5:
Thus,
Thus,
-
{{Displayed math||<math>\frac{1}{2}+i\frac{\sqrt{3}}{2} = 1\cdot \Bigl(\cos\frac{\pi}{3} + i\sin\frac{\pi}{3}\Bigr)</math>}}
+
{{Abgesetzte Formel||<math>\frac{1}{2}+i\frac{\sqrt{3}}{2} = 1\cdot \Bigl(\cos\frac{\pi}{3} + i\sin\frac{\pi}{3}\Bigr)</math>}}
and de Moivre's formula gives
and de Moivre's formula gives
-
{{Displayed math||<math>\begin{align}
+
{{Abgesetzte Formel||<math>\begin{align}
\Bigl(\frac{1}{2}+i\frac{\sqrt{3}}{2}\Bigr)^{12}
\Bigl(\frac{1}{2}+i\frac{\sqrt{3}}{2}\Bigr)^{12}
&= 1^{12}\cdot\Bigl(\cos\Bigl(12\cdot\frac{\pi}{3}\Bigr) + i\sin\Bigl(12\cdot\frac{\pi}{3}\Bigr)\Bigr)\\[5pt]
&= 1^{12}\cdot\Bigl(\cos\Bigl(12\cdot\frac{\pi}{3}\Bigr) + i\sin\Bigl(12\cdot\frac{\pi}{3}\Bigr)\Bigr)\\[5pt]

Version vom 13:10, 10. Mär. 2009

First, we write the number \displaystyle \frac{1}{2}+i\frac{\sqrt{3}}{2} in polar form.

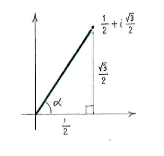
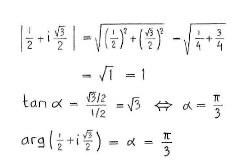
Image:3_3_1_b.gif Image:3_3_1_b_text.gif

Thus,

\displaystyle \frac{1}{2}+i\frac{\sqrt{3}}{2} = 1\cdot \Bigl(\cos\frac{\pi}{3} + i\sin\frac{\pi}{3}\Bigr)

and de Moivre's formula gives

\displaystyle \begin{align}

\Bigl(\frac{1}{2}+i\frac{\sqrt{3}}{2}\Bigr)^{12} &= 1^{12}\cdot\Bigl(\cos\Bigl(12\cdot\frac{\pi}{3}\Bigr) + i\sin\Bigl(12\cdot\frac{\pi}{3}\Bigr)\Bigr)\\[5pt] &= 1\cdot (\cos 4\pi + i\sin 4\pi)\\[5pt] &= 1\cdot (1+i\cdot 0)\\[5pt] &= 1\,\textrm{.} \end{align}

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.3:1b“