Lösung 3.3:2c

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
Aktuelle Version (12:38, 14. Okt. 2011) (bearbeiten) (rückgängig)
 
(Der Versionsvergleich bezieht 11 dazwischen liegende Versionen mit ein.)
Zeile 1: Zeile 1:
-
We write
+
Wir bringen <math>z</math> und <math>-1-i</math> in Polarform.
-
<math>z\text{ }</math>
+
-
and the right-hand side
+
-
<math>\text{-1-}i</math>
+
-
in polar form
+
 +
{{Abgesetzte Formel||<math>\begin{align}
 +
z &= r(\cos\alpha + i\sin\alpha)\,\\[5pt]
 +
-1-i &= \sqrt{2}\Bigl(\cos\frac{5\pi}{4} + i\sin\frac{5\pi}{4}\Bigr)
 +
\end{align}</math>}}
-
<math>\begin{align}
+
Mit den Moivreschen Gesetz erhalten wir die Gleichung
-
& z=r\left( \cos \alpha +i\sin \alpha \right) \\
+
-
& \text{-1-}i=\sqrt{2}\left( \cos \frac{5\pi }{4}+i\sin \frac{5\pi }{4} \right) \\
+
-
\end{align}</math>
+
 +
{{Abgesetzte Formel||<math>r^5(\cos 5\alpha + i\sin 5\alpha) = \sqrt{2}\Bigl(\cos \frac{5\pi}{4} + i\sin\frac{5\pi}{4}\Bigr)\,\textrm{.}</math>}}
-
Using de Moivre's formula, the equation can now be written as
+
Wir vergleichen den Betrag und das Argument der beiden Seiten und erhalten
 +
{{Abgesetzte Formel||<math>\left\{\begin{align}
 +
r^5 &= \sqrt{2}\,,\\[5pt]
 +
5\alpha &= \frac{5\pi}{4} + 2n\pi\,,\quad\text{(n ist eine beliebige ganze Zahl).}
 +
\end{align}\right.</math>}}
-
<math>r^{5}\left( \cos 5\alpha +i\sin 5\alpha \right)=\sqrt{2}\left( \cos \frac{5\pi }{4}+i\sin \frac{5\pi }{4} \right)</math>
 
 +
Die Argumente <math>5\alpha</math> und <math>5\pi/4</math> können sich mit einem Vielfachen von <math>2\pi</math> unterscheiden und trotzdem derselben komplexen Zahl entsprechen.
-
If we identify the magnitude and argument on both sides, we get
+
Wir erhalten also
 +
{{Abgesetzte Formel||<math>\left\{\begin{align}
 +
r &= \sqrt[5]{\sqrt{2}} = \bigl(2^{1/2}\bigr)^{1/5} = 2^{1/10}\,,\\[5pt]
 +
\alpha &= \frac{1}{5}\Bigl(\frac{5\pi}{4}+2n\pi\Bigr) = \frac{\pi}{4} + \frac{2n\pi}{5}\,,\quad\text{(n ist eine beliebige ganze Zahl).}
 +
\end{align}\right.</math>}}
-
<math>\left\{ \begin{array}{*{35}l}
+
Wir sehen, dass das Argument <math>\alpha</math> nur 5 verschiedene Werte annimmt
-
r^{5}=\sqrt{2} \\
+
-
5\alpha =\frac{5\pi }{4}+2n\pi \quad \left( n\text{ an arbitrary integer} \right)\text{ } \\
+
-
\end{array} \right.</math>
+
 +
{{Abgesetzte Formel||<math>\frac{\pi}{4}</math>, <math>\quad\frac{\pi}{4}+\frac{2\pi}{5}</math>, <math>\quad\frac{\pi}{4}+\frac{4\pi}{5}</math>, <math>\quad\frac{\pi}{4}+\frac{6\pi}{5}\quad</math> und <math>\quad\frac{\pi}{4}+\frac{8\pi}{5}</math>,}}
-
(The arguments
+
da sich die Winkel dann wiederholen.
-
<math>5\alpha </math>
+
-
and
+
-
<math>\frac{5\pi }{4}</math>
+
-
can differ by a multiple of
+
-
<math>2\pi </math>
+
-
and still correspond to the same complex number.)
+
-
This gives that
+
Die Wurzeln sind also
 +
{{Abgesetzte Formel||<math>z = 2^{1/10}\,\Bigl(\cos\Bigl(\frac{\pi}{4}+\frac{2n\pi}{5}\Bigr) + i\sin\Bigl(\frac{\pi}{4}+\frac{2n\pi}{5}\Bigr)\Bigr)\,,</math>}}
-
<math>\left\{ \begin{array}{*{35}l}
+
für <math>n=0</math>, <math>1</math>, <math>2</math>, <math>3</math> und
-
r=\sqrt[5]{2}=\left( 2^{{1}/{2}\;} \right)^{{1}/{5}\;}=2^{{1}/{10}\;} \\
+
<math>4</math>.
-
\alpha =\frac{1}{5}\left( \frac{5\pi }{4}+2n\pi \right)=\frac{\pi }{4}+\frac{2n\pi }{5}\quad \left( n\text{ an arbitrary integer} \right)\text{ } \\
+
-
\end{array} \right.</math>
+
 +
[[Image:3_3_2_c.gif|center]]
-
If we investigate the argument
+
Alernativer Lösungsweg: [[3.3:2c_alternativ_exp|Exponentialdarstellung]]
-
<math>\alpha </math>
+
-
more closely, we see that it assumes essentially only five different values,
+
-
 
+
-
 
+
-
<math>\frac{\pi }{4},\ \frac{\pi }{4}+\frac{2\pi }{5},\ \frac{\pi }{4}+\frac{4\pi }{5},\ \frac{\pi }{4}+\frac{6\pi }{5}</math>
+
-
and
+
-
<math>\ \frac{\pi }{4}+\frac{8\pi }{5}</math>
+
-
 
+
-
 
+
-
since these angle values then repeat to within a multiple of
+
-
<math>2\pi </math>.
+
-
 
+
-
In summary, the roots of the equation are
+
-
 
+
-
 
+
-
<math>z=2^{{1}/{10}\;}\left( \cos \left( \frac{\pi }{4}+\frac{2n\pi }{5} \right)+i\sin \left( \frac{\pi }{4}+\frac{2n\pi }{5} \right) \right)</math>
+
-
 
+
-
 
+
-
for
+
-
<math>n=0,\ 1,\ 2,\ 3</math>
+
-
and
+
-
<math>4</math>
+
-
 
+
-
 
+
-
 
+
-
 
+
-
[[Image:3_3_2_c.gif|center]]
+

Aktuelle Version

Wir bringen \displaystyle z und \displaystyle -1-i in Polarform.

\displaystyle \begin{align}

z &= r(\cos\alpha + i\sin\alpha)\,\\[5pt] -1-i &= \sqrt{2}\Bigl(\cos\frac{5\pi}{4} + i\sin\frac{5\pi}{4}\Bigr) \end{align}

Mit den Moivreschen Gesetz erhalten wir die Gleichung

\displaystyle r^5(\cos 5\alpha + i\sin 5\alpha) = \sqrt{2}\Bigl(\cos \frac{5\pi}{4} + i\sin\frac{5\pi}{4}\Bigr)\,\textrm{.}

Wir vergleichen den Betrag und das Argument der beiden Seiten und erhalten

\displaystyle \left\{\begin{align}

r^5 &= \sqrt{2}\,,\\[5pt] 5\alpha &= \frac{5\pi}{4} + 2n\pi\,,\quad\text{(n ist eine beliebige ganze Zahl).} \end{align}\right.


Die Argumente \displaystyle 5\alpha und \displaystyle 5\pi/4 können sich mit einem Vielfachen von \displaystyle 2\pi unterscheiden und trotzdem derselben komplexen Zahl entsprechen.

Wir erhalten also

\displaystyle \left\{\begin{align}

r &= \sqrt[5]{\sqrt{2}} = \bigl(2^{1/2}\bigr)^{1/5} = 2^{1/10}\,,\\[5pt] \alpha &= \frac{1}{5}\Bigl(\frac{5\pi}{4}+2n\pi\Bigr) = \frac{\pi}{4} + \frac{2n\pi}{5}\,,\quad\text{(n ist eine beliebige ganze Zahl).} \end{align}\right.

Wir sehen, dass das Argument \displaystyle \alpha nur 5 verschiedene Werte annimmt

\displaystyle \frac{\pi}{4}, \displaystyle \quad\frac{\pi}{4}+\frac{2\pi}{5}, \displaystyle \quad\frac{\pi}{4}+\frac{4\pi}{5}, \displaystyle \quad\frac{\pi}{4}+\frac{6\pi}{5}\quad und \displaystyle \quad\frac{\pi}{4}+\frac{8\pi}{5},

da sich die Winkel dann wiederholen.

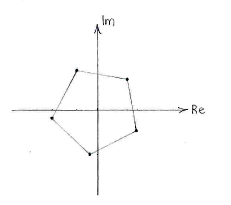
Die Wurzeln sind also

\displaystyle z = 2^{1/10}\,\Bigl(\cos\Bigl(\frac{\pi}{4}+\frac{2n\pi}{5}\Bigr) + i\sin\Bigl(\frac{\pi}{4}+\frac{2n\pi}{5}\Bigr)\Bigr)\,,

für \displaystyle n=0, \displaystyle 1, \displaystyle 2, \displaystyle 3 und \displaystyle 4.

Alernativer Lösungsweg: Exponentialdarstellung

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.3:2c“