Lösung 1.1:4

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
Zeile 1: Zeile 1:
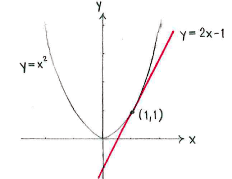
If we write the equation of the tangent as
If we write the equation of the tangent as
 +
{{Displayed math||<math>y=kx+m</math>}}
-
<math>y=kx+m</math>
+
we know that the tangent's slope ''k'' is equal to the derivative of <math>y = x^2</math> at the point <math>x=1\,</math>, and since <math>y^{\,\prime} = 2x\,</math>, so
 +
{{Displayed math||<math>k = y^{\,\prime}(1) = 2\cdot 1 = 2\,\textrm{.}</math>}}
-
we know that the tangent's gradient
+
We can determine the constant ''m'' with the condition that the tangent should go through the grazing point (1,1), i.e. the point (1,1) should satisfy the equation of the tangent
-
<math>k</math>
+
-
is equal to the derivative of
+
-
<math>y=x^{\text{2}}\text{ }</math>
+
-
at the point
+
-
<math>x=\text{1}</math>, and since
+
-
<math>{y}'=\text{2}x</math>, so
+
{{Displayed math||<math>1 = 2\cdot 1 + m</math>}}
-
 
+
-
 
+
-
<math>k={y}'\left( 1 \right)=2\centerdot 1=2</math>
+
-
 
+
-
We can determine the constant
+
-
<math>m\text{ }</math>
+
-
with the condition that the tangent should go through the grazing point
+
-
<math>\left( 1 \right.,\left. 1 \right)</math>, i.e. the point
+
-
<math>\left( 1 \right.,\left. 1 \right)</math>
+
-
should satisfy the equation of the tangent
+
-
 
+
-
 
+
-
<math>1=2\centerdot 1+m</math>
+
-
 
+
-
 
+
-
which gives that
+
-
<math>m=-\text{1}</math>
+
 +
which gives that <math>m=-1</math>.
[[Image:1_1_4_1.gif|center]]
[[Image:1_1_4_1.gif|center]]
 +
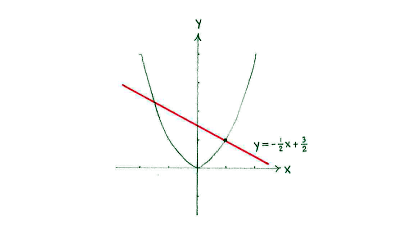
The normal to the curve <math>y=x^2</math> at the point (1,1) is the straight line which is perpendicular to the tangent at the same point.
-
The normal to the curve
+
Because two straight lines which are perpendicular to each other have slopes which satisfy <math>k_{1}\cdot k_{2} = -1\,</math>, the normal must have a slope which is equal to
-
<math>y=x^{\text{2}}\text{ }</math>
+
-
at the point
+
-
<math>\left( 1 \right.,\left. 1 \right)</math>
+
-
is the straight line which is perpendicular to the tangent at the same point.
+
-
 
+
-
Because two straight lines which are perpendicular to each other have gradients which satisfy
+
-
<math>k_{1}\centerdot k_{2}=-1</math>, the normal must have a gradient which is equal to
+
-
 
+
-
 
+
-
<math>-\frac{1}{k}=-\frac{1}{2}</math>
+
 +
{{Displayed math||<math>-\frac{1}{k} = -\frac{1}{2}\,\textrm{.}</math>}}
The equation of the normal can therefore be written as
The equation of the normal can therefore be written as
 +
{{Displayed math||<math>y=-\frac{1}{2}x+n</math>}}
-
<math>y=-\frac{1}{2}x+n</math>
+
where ''n'' is some constant.
-
 
+
-
 
+
-
where
+
-
<math>n</math>
+
-
is some constant.
+
-
 
+
-
Since the normal must pass through the line
+
-
<math>\left( 1 \right.,\left. 1 \right)</math>, we can determine the constant
+
-
<math>n</math>
+
-
if we substitute the point into the equation of the normal,
+
-
 
+
-
 
+
-
<math>1=-\frac{1}{2}\centerdot +n</math>
+
-
 
+
-
and this gives
+
Since the normal must pass through the line (1,1), we can determine the constant ''n'' if we substitute the point into the equation of the normal,
-
<math>n=\frac{3}{2}</math>.
+
 +
{{Displayed math||<math>1=-\frac{1}{2}\cdot + n</math>}}
 +
and this gives <math>n=\tfrac{3}{2}\,</math>.
[[Image:1_1_4-3(3).gif|center]]
[[Image:1_1_4-3(3).gif|center]]

Version vom 13:11, 14. Okt. 2008

If we write the equation of the tangent as

\displaystyle y=kx+m

we know that the tangent's slope k is equal to the derivative of \displaystyle y = x^2 at the point \displaystyle x=1\,, and since \displaystyle y^{\,\prime} = 2x\,, so

\displaystyle k = y^{\,\prime}(1) = 2\cdot 1 = 2\,\textrm{.}

We can determine the constant m with the condition that the tangent should go through the grazing point (1,1), i.e. the point (1,1) should satisfy the equation of the tangent

\displaystyle 1 = 2\cdot 1 + m

which gives that \displaystyle m=-1.

The normal to the curve \displaystyle y=x^2 at the point (1,1) is the straight line which is perpendicular to the tangent at the same point.

Because two straight lines which are perpendicular to each other have slopes which satisfy \displaystyle k_{1}\cdot k_{2} = -1\,, the normal must have a slope which is equal to

\displaystyle -\frac{1}{k} = -\frac{1}{2}\,\textrm{.}

The equation of the normal can therefore be written as

\displaystyle y=-\frac{1}{2}x+n

where n is some constant.

Since the normal must pass through the line (1,1), we can determine the constant n if we substitute the point into the equation of the normal,

\displaystyle 1=-\frac{1}{2}\cdot + n

and this gives \displaystyle n=\tfrac{3}{2}\,.

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_1.1:4“