Aus Online Mathematik Brückenkurs 2

We write \displaystyle z and the right-hand side \displaystyle -1-i in polar form

\displaystyle \begin{align} z &= r(\cos\alpha + i\sin\alpha)\,,\\[5pt] -1-i &= \sqrt{2}\Bigl(\cos\frac{5\pi}{4} + i\sin\frac{5\pi}{4}\Bigr)\,\textrm{.} \end{align}

Using de Moivre's formula, the equation can now be written as

\displaystyle r^5(\cos 5\alpha + i\sin 5\alpha) = \sqrt{2}\Bigl(\cos \frac{5\pi}{4} + i\sin\frac{5\pi}{4}\Bigr)\,\textrm{.}

If we identify the magnitude and argument on both sides, we get

\displaystyle \left\{\begin{align} r^5 &= \sqrt{2}\,,\\[5pt] 5\alpha &= \frac{5\pi}{4} + 2n\pi\,,\quad\text{(n is an arbitrary integer).} \end{align}\right.

(The arguments \displaystyle 5\alpha and \displaystyle 5\pi/4 can differ by a multiple of \displaystyle 2\pi and still correspond to the same complex number.)

This gives that

\displaystyle \left\{\begin{align} r &= \sqrt[5]{2} = \bigl(2^{1/2}\bigr)^{1/5} = 2^{1/10}\,,\\[5pt] \alpha &= \frac{1}{5}\Bigl(\frac{5\pi}{4}+2n\pi\Bigr) = \frac{\pi}{4} + \frac{2n\pi}{5}\,,\quad\text{(n is an arbitrary integer).} \end{align}\right.

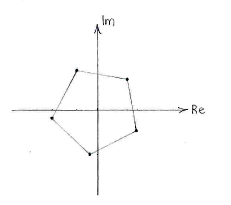
If we investigate the argument \displaystyle \alpha more closely, we see that it assumes essentially only five different values,

\displaystyle \frac{\pi}{4}, \displaystyle \quad\frac{\pi}{4}+\frac{2\pi}{5}, \displaystyle \quad\frac{\pi}{4}+\frac{4\pi}{5}, \displaystyle \quad\frac{\pi}{4}+\frac{6\pi}{5}\quad and \displaystyle \quad\frac{\pi}{4}+\frac{8\pi}{5}

since these angle values then repeat to within a multiple of \displaystyle 2\pi.

In summary, the solutions are

\displaystyle z = 2^{1/10}\,\Bigl(\cos\Bigl(\frac{\pi}{4}+\frac{2n\pi}{5}\Bigr) + i\sin\Bigl(\frac{\pi}{4}+\frac{2n\pi}{5}\Bigr)\Bigr)\,,

for \displaystyle n=0, \displaystyle 1, \displaystyle 2, \displaystyle 3 and \displaystyle 4.