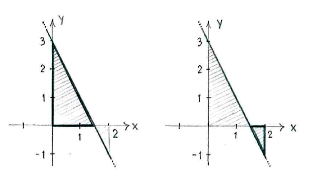Aus Online Mathematik Brückenkurs 2

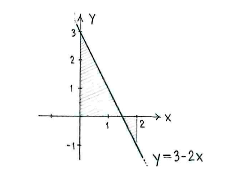
Die Gerade \displaystyle y=3-2x schneidet die x-Achse im Punkt

\displaystyle y=3-2x=0\quad \Leftrightarrow \quad x=3/2

Also liegt ein Teil der Geraden \displaystyle x=3/2 unter der y-Achse.

Wenn wir das Integral berechen, müssen wir berücksichtigen dass die Fläche die unter der y-Achse liegt, von der Fläche oberhalb der y-Achse subtrahiert werden muss.

Wir teilen unsere Fläche also auf,

If we divide up the area between the straight line and the x-axis at \displaystyle x=3/2, we see that the value of the integral is the area of the triangle to the left in the figure below, minus the area of the triangle to the right.

und erhalten

\displaystyle \int\limits_{0}^{2} (3-2x)\,dx = \frac{1}{2}\cdot\frac{3}{2}\cdot 3 - \frac{1}{2}\cdot\frac{1}{2}\cdot 1 = \frac{9}{4} - \frac{1}{4} = 2\,\textrm{.}