Aus Online Mathematik Brückenkurs 2

An equation of the type “ \displaystyle z^{n} = a complex number” is called a binomial equation and these are usually solved by going over to polar form and using de Moivre's formula.

We start by writing \displaystyle z\text{ } and \displaystyle \text{1} in polar form

\displaystyle \begin{align} & z=r\left( \cos \alpha +i\sin \alpha \right) \\ & 1=1\left( \cos 0+i\sin 0 \right) \\ \end{align}

The equation then becomes

\displaystyle r^{4}\left( \cos 4\alpha +i\sin 4\alpha \right)=1\left( \cos 0+i\sin 0 \right)

where we have used de Moivre's formula on the left-hand side. In order that both sides are equal, they must have the same magnitude and the same argument to within a multiple of \displaystyle 2\pi , i.e.

\displaystyle \left\{ \begin{array}{*{35}l} r^{4}=1 \\ 4\alpha =0+2n\pi \quad \left( n\text{ an arbitrary integer} \right)\text{ } \\ \end{array} \right.

This means that

\displaystyle \left\{ \begin{array}{*{35}l} r=1 \\ \alpha =\frac{n\pi }{2}\quad \left( n\text{ an arbitrary integer} \right)\text{ } \\ \end{array} \right.

The solutions are thus (in polar form)

\displaystyle z=1\centerdot \left( \cos \frac{n\pi }{2}+i\sin \frac{n\pi }{2} \right), for \displaystyle n=0,\ \pm 1,\ \pm 2,...

but observe that the argument on the right-hand side essentially takes only four different values \displaystyle 0,\ {\pi }/{2}\;,\ \pi and \displaystyle {3\pi }/{2}\;, because other values of \displaystyle n\text{ } give some of these values plus/minus a multiple of \displaystyle 2\pi .

The equation's solutions are therefore

\displaystyle z=\left\{ \begin{array}{*{35}l} 1\centerdot \left( \cos 0+i\sin 0 \right) \\ 1\centerdot \left( \cos {\pi }/{2}\;+i\sin {\pi }/{2}\; \right) \\ 1\centerdot \left( \cos \pi +i\sin \pi \right) \\ 1\centerdot \left( \cos {3\pi }/{2}\;+i\sin {3\pi }/{2}\; \right) \\ \end{array} \right.=\left\{ \begin{matrix} 1 \\ i \\ -1 \\ -i \\ \end{matrix} \right.

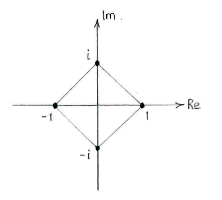
NOTE: note that if we mark these solutions on the complex number plane, we see that they are corners in a regular quadrilateral.