Lösung 3.2:2e

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Robot: Automated text replacement (-ä +ä))
Zeile 1: Zeile 1:
-
Wir schreiben die gesuchte komplexe Zahl <math> z </math> mit ihrem Realteil und Imagin&auml;rteil. Nach Aufgabenstellung ist der Realteil <math> x </math>, den unbekannten Imagin&auml;rteil nennen wir <math> y </math>.
+
Wir schreiben die gesuchte komplexe Zahl <math> z </math> mit ihrem Realteil und Imaginärteil. Nach Aufgabenstellung ist der Realteil <math> x </math>, den unbekannten Imaginärteil nennen wir <math> y </math>.
{{Abgesetzte Formel||<math>z = x + i y </math>.}}
{{Abgesetzte Formel||<math>z = x + i y </math>.}}
Zeile 9: Zeile 9:
Also soll gelten
Also soll gelten
{{Abgesetzte Formel||<math> x = x + i (1-y) </math>}}
{{Abgesetzte Formel||<math> x = x + i (1-y) </math>}}
-
und das ist &auml;quivalent zu
+
und das ist äquivalent zu
{{Abgesetzte Formel||<math> 0 = i (1-y) </math>.}}
{{Abgesetzte Formel||<math> 0 = i (1-y) </math>.}}

Version vom 10:35, 16. Sep. 2009

Wir schreiben die gesuchte komplexe Zahl \displaystyle z mit ihrem Realteil und Imaginärteil. Nach Aufgabenstellung ist der Realteil \displaystyle x , den unbekannten Imaginärteil nennen wir \displaystyle y .

\displaystyle z = x + i y .

Es soll also gelten

\displaystyle x = i + \overline{z} = i + (x -iy) = x + i (1-y) .

Also soll gelten

\displaystyle x = x + i (1-y)

und das ist äquivalent zu

\displaystyle 0 = i (1-y) .

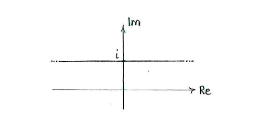
daher ist \displaystyle y=1.

Also besteht unsere Lösungsmege aus allen komlexen Zahlen deren Imaginärteil 1 ist.

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.2:2e“