Lösung 3.2:6c
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K (Solution 3.2:6c moved to Lösung 3.2:6c: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
| - | + | Wir brauchen nur den Betrag und das Argument um die Zahl auf Polarform zu bringen. | |
| - | + | Den betrag erhalten wir durch die Formel | |
{{Abgesetzte Formel||<math>|-4-4i| = \sqrt{(-4)^2+(-4)^2} = \sqrt{16+16} = \sqrt{16\cdot 2} = 4\sqrt{2}\,\textrm{.}</math>}} | {{Abgesetzte Formel||<math>|-4-4i| = \sqrt{(-4)^2+(-4)^2} = \sqrt{16+16} = \sqrt{16\cdot 2} = 4\sqrt{2}\,\textrm{.}</math>}} | ||
| - | + | Weiterhin lieht die Zahl in dritten Quadrant, und definieren wir ein Dreieck wie in der Figur, können wir zuerst den Winkel <math>\alpha</math> bestimmen. Addieren wir <math>\pi</math> zu diesen Winkel, erhalten wir das Argument. | |
<center>[[Image:3_2_6_c1.gif]] [[Image:3_2_6_c2.gif]]</center> | <center>[[Image:3_2_6_c1.gif]] [[Image:3_2_6_c2.gif]]</center> | ||
| - | + | Die Polarform der Zahl ist daher | |
{{Abgesetzte Formel||<math>4\sqrt{2}\Bigl(\cos\frac{5\pi}{4} + i\sin\frac{5\pi}{4} \Bigr)\,\textrm{.}</math>}} | {{Abgesetzte Formel||<math>4\sqrt{2}\Bigl(\cos\frac{5\pi}{4} + i\sin\frac{5\pi}{4} \Bigr)\,\textrm{.}</math>}} | ||
Version vom 13:09, 13. Mai 2009
Wir brauchen nur den Betrag und das Argument um die Zahl auf Polarform zu bringen.
Den betrag erhalten wir durch die Formel
| \displaystyle |-4-4i| = \sqrt{(-4)^2+(-4)^2} = \sqrt{16+16} = \sqrt{16\cdot 2} = 4\sqrt{2}\,\textrm{.} |
Weiterhin lieht die Zahl in dritten Quadrant, und definieren wir ein Dreieck wie in der Figur, können wir zuerst den Winkel \displaystyle \alpha bestimmen. Addieren wir \displaystyle \pi zu diesen Winkel, erhalten wir das Argument.
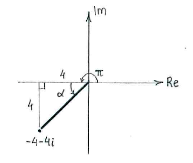
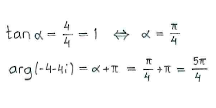
Die Polarform der Zahl ist daher
| \displaystyle 4\sqrt{2}\Bigl(\cos\frac{5\pi}{4} + i\sin\frac{5\pi}{4} \Bigr)\,\textrm{.} |
