Lösung 1.3:4

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Solution 1.3:4 moved to Lösung 1.3:4: Robot: moved page)
Zeile 1: Zeile 1:
-
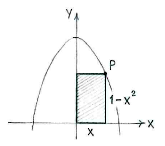
If we call the ''x''-coordinate of the point <math>P</math> <math>x</math>, then its ''y''-coordinate is <math>1-x^{2}</math>, because <math>P</math> lies on the curve <math>y=1-x^{2}</math>.
+
Wir benennen die ''x''-Koordinate des Punktes <math>P</math> <math>x</math>. Die ''y''Koordinate ist dann <math>1-x^{2}</math>, nachdem <math>P</math> auf der Kurve <math>y=1-x^{2}</math> liegt.
[[Image:1_3_4-1-1.gif|center]]
[[Image:1_3_4-1-1.gif|center]]
-
The area of the rectangle is then given by
+
Die Fläche des Rechteckes ist
-
{{Abgesetzte Formel||<math>A(x) = \text{(base)}\cdot\text{(height)} = x\cdot (1-x^2)</math>}}
+
{{Abgesetzte Formel||<math>A(x) = \text{(Basis)}\cdot\text{(Höhe)} = x\cdot (1-x^2)</math>}}
-
and we will try to choose <math>x</math> so that this area function is maximised.
+
Wir wollen diese Fläche in Bezug auf ''x'' maximieren.
-
To begin with, we note that, because <math>P</math> should lie in the first quadrant, <math>x\ge 0</math> and also <math>y=1-x^2\ge 0</math>, i.e. <math>x\le 1</math>. We should therefore look for the maximum of <math>A(x)</math> when <math>0\le x\le 1\,</math>.
+
Wir sehen von der Figur her dass <math>P</math> in der ersten Quadrante liegen muss, <math>x\ge 0</math>, und also <math>y=1-x^2\ge 0</math>, und wir erhalten dass <math>x\le 1</math>. Also suchen wir das Maxima von<math>A(x)</math> im Bereich <math>0\le x\le 1\,</math>.
-
There are three types of points which can maximise the area function:
 
-
# critical points,
+
Lokale Extrempunkte der Fläche sind entweder:
-
# points where the function is not differentiable,
+
-
# endpoints of the region of definition.
+
-
The function <math>A(x) = x(1-x^2)</math> is differentiable everywhere, so item 2 does not apply. In addition, <math>A(0) = A(1) = 0\,</math>, so the endpoints in item 3 cannot be maximum points (but rather the opposite, i.e. minimum points).
+
# stationäre Punkte, wo <math>f^{\,\prime}(x)=0</math>,
 +
# Singuläre Punkte, wo die Funktion nicht ableitbar ist, oder
 +
# Endpunkte.
 +
Die Funktion <math>A(x) = x(1-x^2)</math> ist überall differenzierbar, so der wir müssen den 2:en Fall nicht beachten. Die Endpunkte <math>A(0) = A(1) = 0\,</math> können aber lokale Extrempunkte sein (offenbar lokale Minima, siehe Figur).
-
We must therefore conclude that the maximum area is a critical point. We differentiate
+
Die Ableitung der Funktion ist
{{Abgesetzte Formel||<math>A'(x) = 1\cdot (1-x^2) + x\cdot (-2x) = 1-3x^2\,,</math>}}
{{Abgesetzte Formel||<math>A'(x) = 1\cdot (1-x^2) + x\cdot (-2x) = 1-3x^2\,,</math>}}
-
and the condition that the derivative should be zero gives that <math>x=\pm 1/\!\sqrt{3}</math>; however, it is only <math>x=1/\!\sqrt{3}</math> which satisfies <math>0\le x\le 1</math>.
+
und wir erhalten die Gleichung <math>x=\pm 1/\!\sqrt{3}</math> für die stationären Punkte. Nur die Lösung <math>x=1/\!\sqrt{3}</math> erfüllt aber <math>0\le x\le 1</math>.
-
At the critical point, the second derivative <math>A''(x)=-6x</math> has the value
+
Die zweite Ableitung <math>A''(x)=-6x</math> hat im stationären Punkt den Wert
{{Abgesetzte Formel||<math>A''\bigl( 1/\!\sqrt{3}\bigr) = -6\cdot\frac{1}{\sqrt{3}} < 0\,,</math>}}
{{Abgesetzte Formel||<math>A''\bigl( 1/\!\sqrt{3}\bigr) = -6\cdot\frac{1}{\sqrt{3}} < 0\,,</math>}}
-
which shows that <math>x=1/\!\sqrt{3}</math> is a local maximum.
+
und also ist <math>x=1/\!\sqrt{3}</math> ein lokales Maxima.
-
The answer is that the point <math>P</math> should be chosen so that
+
Also ist der optimale Punkt <math>P</math>:
{{Abgesetzte Formel||<math>P = \Bigl(\frac{1}{\sqrt{3}}, 1-\Bigl(\frac{1}{\sqrt{3}} \Bigr)^2\, \Bigr) = \Bigl(\frac{1}{\sqrt{3}}, \frac{2}{3} \Bigr)\,\textrm{.}</math>}}
{{Abgesetzte Formel||<math>P = \Bigl(\frac{1}{\sqrt{3}}, 1-\Bigl(\frac{1}{\sqrt{3}} \Bigr)^2\, \Bigr) = \Bigl(\frac{1}{\sqrt{3}}, \frac{2}{3} \Bigr)\,\textrm{.}</math>}}

Version vom 11:39, 27. Apr. 2009

Wir benennen die x-Koordinate des Punktes \displaystyle P \displaystyle x. Die yKoordinate ist dann \displaystyle 1-x^{2}, nachdem \displaystyle P auf der Kurve \displaystyle y=1-x^{2} liegt.

Die Fläche des Rechteckes ist

\displaystyle A(x) = \text{(Basis)}\cdot\text{(Höhe)} = x\cdot (1-x^2)

Wir wollen diese Fläche in Bezug auf x maximieren.

Wir sehen von der Figur her dass \displaystyle P in der ersten Quadrante liegen muss, \displaystyle x\ge 0, und also \displaystyle y=1-x^2\ge 0, und wir erhalten dass \displaystyle x\le 1. Also suchen wir das Maxima von\displaystyle A(x) im Bereich \displaystyle 0\le x\le 1\,.


Lokale Extrempunkte der Fläche sind entweder:

  1. stationäre Punkte, wo \displaystyle f^{\,\prime}(x)=0,
  2. Singuläre Punkte, wo die Funktion nicht ableitbar ist, oder
  3. Endpunkte.

Die Funktion \displaystyle A(x) = x(1-x^2) ist überall differenzierbar, so der wir müssen den 2:en Fall nicht beachten. Die Endpunkte \displaystyle A(0) = A(1) = 0\, können aber lokale Extrempunkte sein (offenbar lokale Minima, siehe Figur).

Die Ableitung der Funktion ist

\displaystyle A'(x) = 1\cdot (1-x^2) + x\cdot (-2x) = 1-3x^2\,,

und wir erhalten die Gleichung \displaystyle x=\pm 1/\!\sqrt{3} für die stationären Punkte. Nur die Lösung \displaystyle x=1/\!\sqrt{3} erfüllt aber \displaystyle 0\le x\le 1.

Die zweite Ableitung \displaystyle A''(x)=-6x hat im stationären Punkt den Wert

\displaystyle A''\bigl( 1/\!\sqrt{3}\bigr) = -6\cdot\frac{1}{\sqrt{3}} < 0\,,

und also ist \displaystyle x=1/\!\sqrt{3} ein lokales Maxima.

Also ist der optimale Punkt \displaystyle P:

\displaystyle P = \Bigl(\frac{1}{\sqrt{3}}, 1-\Bigl(\frac{1}{\sqrt{3}} \Bigr)^2\, \Bigr) = \Bigl(\frac{1}{\sqrt{3}}, \frac{2}{3} \Bigr)\,\textrm{.}
Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_1.3:4“