Lösung 3.2:6e
Aus Online Mathematik Brückenkurs 2
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
K (Solution 3.2:6e moved to Lösung 3.2:6e: Robot: moved page) |
Version vom 10:41, 11. Mär. 2009
If we manage to write the numerator and denominator in polar form, we can then use the fact that when division is carried out in polar form it is particularly simple. Division means, namely, that the numerator's magnitude is divided by the denominator's magnitude and that the numerator's argument is subtracted from denominator's argument or, in written form,
| \displaystyle \frac{r_1(\cos\alpha+i\sin\alpha)}{r_2(\cos\beta+i\sin\beta)} = \frac{r_1}{r_2}\bigl(\cos (\alpha-\beta) + i\sin (\alpha-\beta)\bigr)\,\textrm{.} |
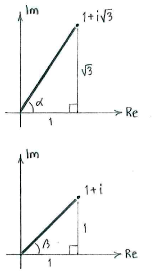
Thus, we determine the numerator's and denominator's polar forms:
Now, we obtain
| \displaystyle \begin{align}
\frac{1+i\sqrt{3}}{1+i} &= \frac{2\Bigl(\cos\dfrac{\pi}{3}+i\sin\dfrac{\pi}{3}\Bigr)}{\sqrt{2}\Bigl(\cos \dfrac{\pi}{4} + i\sin\dfrac{\pi}{4}\Bigr)}\\[5pt] &= \frac{2}{\sqrt{2}}\Bigl( \cos\Bigl(\frac{\pi}{3}-\frac{\pi}{4}\Bigr) + i\sin\Bigl( \frac{\pi}{3}-\frac{\pi}{4}\Bigr)\Bigr)\\[5pt] &= \sqrt{2}\Bigl(\cos\frac{\pi}{12}+i\sin\frac{\pi}{12}\Bigr)\,\textrm{.} \end{align} |