Lösung 1.3:5
Aus Online Mathematik Brückenkurs 2
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
K (Solution 1.3:5 moved to Lösung 1.3:5: Robot: moved page) |
Version vom 10:12, 11. Mär. 2009
The channel holds most water when its cross-sectional area is greatest.
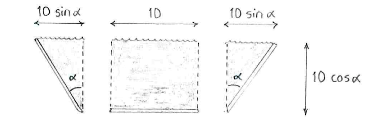
By dividing up the channel's cross-section into a rectangle and two triangles we can, with the help of a little trigonometry, determine the lengths of the rectangle's and triangles' sides, and thence the cross-sectional area.
The area of the cross-section is
| \displaystyle \begin{align}
A(\alpha) &= 10\cdot 10\cos\alpha + 2\cdot\frac{1}{2}\cdot 10\cos\alpha \cdot 10\sin\alpha\\[5pt] &= 100\cos \alpha (1+\sin\alpha)\,\textrm{.} \end{align} |
If we limit the angle to lie between \displaystyle 0 and \displaystyle \pi/2, the problem can be formulated as:
- Maximise the function \displaystyle A(\alpha) = 100\cos\alpha (1+\sin\alpha) when
\displaystyle 0\le \alpha \le {\pi }/{2}\,.
The area function is a differentiable function and the area is least when \displaystyle \alpha=0 or \displaystyle \alpha=\pi/2\,, so the area must assume its maximum at a critical point of the area function.
We differentiate the area function:
| \displaystyle \begin{align}
A'(\alpha) &= 100\cdot (-\sin\alpha)\cdot (1+\sin\alpha) + 100\cdot\cos\alpha \cdot \cos\alpha\\[5pt] &= -100\sin\alpha - 100\sin^2\!\alpha + 100\cos^2\!\alpha\,\textrm{.} \end{align} |
At a critical point \displaystyle A'(\alpha)=0 and this gives us the equation
| \displaystyle \sin\alpha + \sin^2\!\alpha - \cos^2\!\alpha = 0 |
after eliminating the factor -100. We replace \displaystyle \cos^2\!\alpha with \displaystyle 1-\sin^2\!\alpha (according to the Pythagorean identity) to obtain an equation solely in \displaystyle \sin\alpha\,,
| \displaystyle \begin{align}
\sin\alpha + \sin^2\!\alpha - (1-\sin^2\!\alpha) &= 0\\[5pt] 2\sin^2\!\alpha + \sin\alpha - 1 &= 0\,\textrm{.} \end{align} |
This is a second-degree equation in \displaystyle \sin\alpha and completing the square gives that
| \displaystyle \begin{align}
2\bigl(\sin\alpha + \tfrac{1}{4}\bigr)^{2} - 2\bigl(\tfrac{1}{4}\bigr)^2 - 1 &= 0\\[5pt] \bigl(\sin\alpha + \tfrac{1}{4}\bigr)^2 &= \frac{9}{16} \end{align} |
and we obtain \displaystyle \sin\alpha = -\tfrac{1}{4}\pm \tfrac{3}{4}, i.e. \displaystyle \sin \alpha = -1 or \displaystyle \sin \alpha = \tfrac{1}{2}\,.
The case \displaystyle \sin \alpha =-1 is not satisfied for \displaystyle 0\le \alpha \le \pi/2 and \displaystyle \sin \alpha = \tfrac{1}{2} gives \displaystyle \alpha = \pi/6. Thus \displaystyle \alpha = \pi/6\, is a critical point.
If we summarize, we know therefore that the cross-sectional area has local minimum points at \displaystyle \alpha = 0 and \displaystyle \alpha = \pi/2 and that we have a critical point at \displaystyle \alpha = \pi/6\,. This critical point must be a maximum, which we can also show using the second derivative,
| \displaystyle \begin{align}
A''(\alpha) &= -100\cos\alpha - 100\cdot 2\sin\alpha\cdot\cos\alpha + 100\cdot 2\cos\alpha \cdot (-\sin\alpha)\\[5pt] &= -100\cos\alpha (1+4\sin\alpha)\,, \end{align} |
which is negative at \displaystyle \alpha = \pi/6,
| \displaystyle \begin{align}
A''(\pi/6) &= -100\cos\frac{\pi}{6}\cdot \Bigl(1+4\sin\frac{\pi}{6}\Bigr)\\[5pt] &= -100\cdot\frac{\sqrt{3}}{2}\cdot \Bigl( 1+4\cdot \frac{1}{2} \Bigr)<0\,\textrm{.} \end{align} |
There are no local maximum points other than \displaystyle \alpha = \pi/6\,, which must therefore also be a global maximum.