Lösung 3.2:4a

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel))
Zeile 3: Zeile 3:
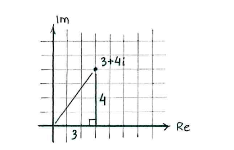
If we treat the line from the origin to <math>3+4i</math> as the hypotenuse in a right-angled triangle which has its legs parallel with the real and imaginary axes, then the Pythagorean theorem gives that the magnitude is
If we treat the line from the origin to <math>3+4i</math> as the hypotenuse in a right-angled triangle which has its legs parallel with the real and imaginary axes, then the Pythagorean theorem gives that the magnitude is
-
{{Displayed math||<math>|3+4i| = \sqrt{3^2+4^2} = \sqrt{9+16} = \sqrt{25} = 5\,\textrm{.}</math>}}
+
{{Abgesetzte Formel||<math>|3+4i| = \sqrt{3^2+4^2} = \sqrt{9+16} = \sqrt{25} = 5\,\textrm{.}</math>}}
[[Image:3_2_4_a.gif|center]]
[[Image:3_2_4_a.gif|center]]
Zeile 10: Zeile 10:
Note: In general, the magnitude of a complex number <math>z=x+iy</math> is equal to
Note: In general, the magnitude of a complex number <math>z=x+iy</math> is equal to
-
{{Displayed math||<math>|z| = |x+iy| = \sqrt{x^2+y^2}\,\textrm{.}</math>}}
+
{{Abgesetzte Formel||<math>|z| = |x+iy| = \sqrt{x^2+y^2}\,\textrm{.}</math>}}

Version vom 13:08, 10. Mär. 2009

The magnitude of the number \displaystyle 3+4i is the number's distance to the origin in the complex number plane.

If we treat the line from the origin to \displaystyle 3+4i as the hypotenuse in a right-angled triangle which has its legs parallel with the real and imaginary axes, then the Pythagorean theorem gives that the magnitude is

\displaystyle |3+4i| = \sqrt{3^2+4^2} = \sqrt{9+16} = \sqrt{25} = 5\,\textrm{.}


Note: In general, the magnitude of a complex number \displaystyle z=x+iy is equal to

\displaystyle |z| = |x+iy| = \sqrt{x^2+y^2}\,\textrm{.}
Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.2:4a“