Lösung 3.2:2e

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel))
Zeile 6: Zeile 6:
and the condition becomes
and the condition becomes
-
{{Displayed math||<math>x=x+(1-y)i\quad\Leftrightarrow\quad 0=(1-y)i</math>}}
+
{{Abgesetzte Formel||<math>x=x+(1-y)i\quad\Leftrightarrow\quad 0=(1-y)i</math>}}
which means that <math>y=1</math>.
which means that <math>y=1</math>.

Version vom 13:08, 10. Mär. 2009

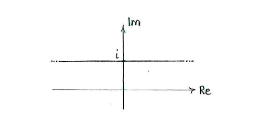
Because the expression contains both \displaystyle z and \displaystyle \bar{z}, we write out \displaystyle z=x+iy, where \displaystyle x is the real part of \displaystyle z and \displaystyle y is the imaginary part. Thus, we have

  • \displaystyle \mathop{\rm Re}z = x
  • \displaystyle i+\bar{z} = i+(x-iy) = x+(1-y)i

and the condition becomes

\displaystyle x=x+(1-y)i\quad\Leftrightarrow\quad 0=(1-y)i

which means that \displaystyle y=1.

The set therefore consists of all complex numbers with imaginary part 1.

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.2:2e“