Lösung 2.2:2a
Aus Online Mathematik Brückenkurs 2
K |
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
||
| Zeile 1: | Zeile 1: | ||
The integral is a standard integral, with <math>5x</math> as the argument of the cosine function. If we therefore substitute <math>u=5x</math>, we obtain the “correct” argument of the cosine, | The integral is a standard integral, with <math>5x</math> as the argument of the cosine function. If we therefore substitute <math>u=5x</math>, we obtain the “correct” argument of the cosine, | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\int\limits_0^{\pi} \cos 5x\,dx = \left\{\begin{align} |
u &= 5x\\[5pt] | u &= 5x\\[5pt] | ||
du &= (5x)'\,dx = 5\,dx | du &= (5x)'\,dx = 5\,dx | ||
| Zeile 11: | Zeile 11: | ||
Now, we have a standard integral which we can easily compute, | Now, we have a standard integral which we can easily compute, | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\frac{1}{5}\int\limits_0^{5\pi} \cos u\,du = \frac{1}{5}\Bigl[\ \sin u\ \Bigr]_0^{5\pi} = \frac{1}{5}( \sin 5\pi -\sin 0) = \frac{1}{5}(0-0) = 0\,\textrm{.}</math>}} |
Version vom 13:01, 10. Mär. 2009
The integral is a standard integral, with \displaystyle 5x as the argument of the cosine function. If we therefore substitute \displaystyle u=5x, we obtain the “correct” argument of the cosine,
| \displaystyle \int\limits_0^{\pi} \cos 5x\,dx = \left\{\begin{align}
u &= 5x\\[5pt] du &= (5x)'\,dx = 5\,dx \end{align}\right\} = \frac{1}{5}\int\limits_0^{5\pi} \cos u\,du\,\textrm{.} |
As can be seen, the variable change replaced \displaystyle dx by \displaystyle \tfrac{1}{5}\,du and the new limits of integration become \displaystyle u=5\cdot 0=0 and \displaystyle u=5\cdot \pi = 5\pi\,.
Now, we have a standard integral which we can easily compute,
| \displaystyle \frac{1}{5}\int\limits_0^{5\pi} \cos u\,du = \frac{1}{5}\Bigl[\ \sin u\ \Bigr]_0^{5\pi} = \frac{1}{5}( \sin 5\pi -\sin 0) = \frac{1}{5}(0-0) = 0\,\textrm{.} |
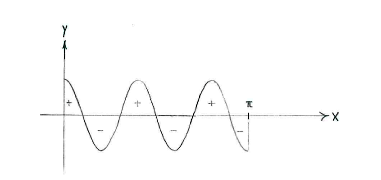
Note: If we draw the graph of \displaystyle y=\cos 5x, we see also that the area between the curve and x-axis above the x-axis is the same as the area under the x-axis.