Lösung 3.3:1a

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
Zeile 1: Zeile 1:
-
Powers are repeated multiplications and because multiplication is a relatively simple arithmetical operation when it is carried out in polar form, calculating powers also becomes fairly simple in polar form:
+
Powers are repeated multiplications and because multiplication is a relatively simple arithmetical operation when it is carried out in polar form, calculating powers also becomes fairly simple in polar form,
-
 
+
-
 
+
-
<math>\left( r\left( \cos \alpha +i\sin \alpha \right) \right)^{n}=r^{n}\left( \cos n\alpha +i\sin n\alpha \right)</math>
+
 +
{{Displayed math||<math>\bigl(r(\cos\alpha + i\sin\alpha)\bigr)^n = r^n(\cos n\alpha + i\sin n\alpha)\,\textrm{.}</math>}}
The equation above is called de Moivre's formula.
The equation above is called de Moivre's formula.
-
The plan is therefore to rewrite
+
The plan is therefore to rewrite <math>1+i</math> in polar form, raise the expression to the power <math>12</math> using de Moivre's formula and then to write the answer in the form <math>a+ib</math>.
-
<math>\text{1}+i</math>
+
-
in polar form, raised the expression to the power
+
-
<math>\text{12}</math>
+
-
using de Moivre's formula and then to write the answer in the form
+
-
<math>a+ib</math>.
+
-
 
+
-
 
+
-
 
+
-
[[Image:3_3_1_a1.gif]] [[Image:3_3_1_a_text.gif]]
+
 +
<center>[[Image:3_3_1_a1.gif]] [[Image:3_3_1_a_text.gif]]</center>
Using the calculations above, we see that
Using the calculations above, we see that
 +
{{Displayed math||<math>1+i = \sqrt{2}\Bigl(\cos\frac{\pi}{4} + i\sin\frac{\pi}{4} \Bigr)\,\textrm{.}</math>}}
-
<math>\text{1}+i=\sqrt{2}\left( \cos \frac{\pi }{4}+i\sin \frac{\pi }{4} \right)</math>
+
De Moivre's formula now gives
-
 
+
-
 
+
-
de Moivre's formula now gives
+
-
 
+
-
<math>\begin{align}
+
{{Displayed math||<math>\begin{align}
-
& \left( \text{1}+i \right)^{12}=\left( \sqrt{2} \right)^{12}\left( \cos 12\centerdot \frac{\pi }{4}+i\sin 12\centerdot \frac{\pi }{4} \right) \\
+
(1+i)^{12}
-
& =2^{\frac{1}{2}\centerdot 12}\left( \cos 3\pi +i\sin 3\pi \right) \\
+
&= \bigl(\sqrt{2}\,\bigr)^{12}\Bigl(\cos \Bigl(12\cdot\frac{\pi}{4}\Bigr) + i\sin \Bigl(12\cdot\frac{\pi}{4}\Bigr)\Bigr)\\[5pt]
-
& =2^{6}\left( -1+i\centerdot 0 \right)=64\centerdot \left( -1 \right)=-64 \\
+
&= 2^{(1/2)\cdot 12}\Bigl(\cos 3\pi + i\sin 3\pi\Bigr)\\[5pt]
-
& \\
+
&= 2^6(-1+i\cdot 0)\\[5pt]
-
\end{align}</math>
+
&= 64\cdot (-1)\\[5pt]
 +
&= -64\,\textrm{.}
 +
\end{align}</math>}}

Version vom 08:50, 30. Okt. 2008

Powers are repeated multiplications and because multiplication is a relatively simple arithmetical operation when it is carried out in polar form, calculating powers also becomes fairly simple in polar form,

\displaystyle \bigl(r(\cos\alpha + i\sin\alpha)\bigr)^n = r^n(\cos n\alpha + i\sin n\alpha)\,\textrm{.}

The equation above is called de Moivre's formula.

The plan is therefore to rewrite \displaystyle 1+i in polar form, raise the expression to the power \displaystyle 12 using de Moivre's formula and then to write the answer in the form \displaystyle a+ib.

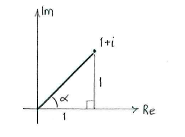
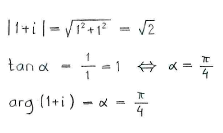
Image:3_3_1_a1.gif Image:3_3_1_a_text.gif

Using the calculations above, we see that

\displaystyle 1+i = \sqrt{2}\Bigl(\cos\frac{\pi}{4} + i\sin\frac{\pi}{4} \Bigr)\,\textrm{.}

De Moivre's formula now gives

\displaystyle \begin{align}

(1+i)^{12} &= \bigl(\sqrt{2}\,\bigr)^{12}\Bigl(\cos \Bigl(12\cdot\frac{\pi}{4}\Bigr) + i\sin \Bigl(12\cdot\frac{\pi}{4}\Bigr)\Bigr)\\[5pt] &= 2^{(1/2)\cdot 12}\Bigl(\cos 3\pi + i\sin 3\pi\Bigr)\\[5pt] &= 2^6(-1+i\cdot 0)\\[5pt] &= 64\cdot (-1)\\[5pt] &= -64\,\textrm{.} \end{align}

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.3:1a“