Lösung 2.1:4e
Aus Online Mathematik Brückenkurs 2
K |
|||
| Zeile 1: | Zeile 1: | ||
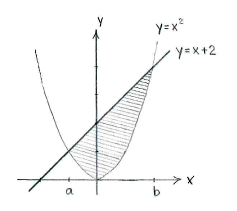
| - | The double inequality means that we look for the area of the region which is bounded above in the y-direction by the straight line | + | The double inequality means that we look for the area of the region which is bounded above in the y-direction by the straight line <math>y=x+2</math> and from below by the parabola <math>y=x^2</math>. |
| - | <math>y=x+ | + | |
| - | and from below by the parabola | + | |
| - | <math>y=x^ | + | |
If we sketch the line and the parabola, the region is given by the region shaded in the figure below. | If we sketch the line and the parabola, the region is given by the region shaded in the figure below. | ||
| - | |||
| - | |||
[[Image:2_1_4_e.gif|center]] | [[Image:2_1_4_e.gif|center]] | ||
| + | As soon as we have determined the ''x''-coordinates of the points of intersection, | ||
| + | <math>x=a</math> and <math>x=b</math>, between the line and the parabola, we can calculate the area as the integral of the difference between the curves' ''y''-values, | ||
| - | + | {{Displayed math||<math>\text{Area} = \int\limits_a^b \bigl(x+2-x^2\bigr)\,dx\,\textrm{.}</math>}} | |
| - | <math>x | + | |
| - | - | + | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | The curves' points of intersection are those points which lie on both curves, i.e. which satisfy both curves' equations | |
| - | + | ||
| + | {{Displayed math||<math>\left\{\begin{align} | ||
| + | y &= x+2\,,\\[5pt] | ||
| + | y &= x^2\,\textrm{.} | ||
| + | \end{align} \right.</math>}} | ||
| - | + | By eliminating <math>y</math>, we obtain an equation for <math>x</math>, | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | By eliminating | + | |
| - | <math>y</math>, we obtain an equation for | + | |
| - | <math>x</math>, | + | |
| + | {{Displayed math||<math>x^{2}=x+2\,\textrm{.}</math>}} | ||
| - | + | If we move all ''x''-terms to the left-hand side, | |
| - | + | ||
| - | + | ||
| - | If we move all x-terms to the left-hand side, | + | |
| - | + | ||
| - | + | ||
| - | + | ||
| + | {{Displayed math||<math>x^2-x=2\,,</math>}} | ||
and complete the square, we obtain | and complete the square, we obtain | ||
| + | {{Displayed math||<math>\begin{align} | ||
| + | \Bigl(x-\frac{1}{2}\Bigr)^2 - \Bigl(\frac{1}{2}\Bigr)^2 &= 2\\[5pt] | ||
| + | \Bigl(x-\frac{1}{2}\Bigr)^2 &= \frac{9}{4}\,\textrm{.} | ||
| + | \end{align}</math>}} | ||
| - | + | Taking the root then gives that <math>x=\tfrac{1}{2}\pm \tfrac{3}{2}</math>. In other words, <math>x=-1</math> and <math>x=2\,</math>. | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | Taking the root then gives that | + | |
| - | <math>x=\ | + | |
| - | <math>x=- | + | |
| - | and | + | |
| - | <math>x=\ | + | |
The area of the region is now given by | The area of the region is now given by | ||
| - | + | {{Displayed math||<math>\begin{align} | |
| - | <math>\begin{align} | + | \text{Area} |
| - | + | &= \int\limits_{-1}^2 \bigl(x+2-x^2\bigr)\,dx\\[5pt] | |
| - | & =\frac{2^ | + | &= \Bigl[\ \frac{x^2}{2} + 2x - \frac{x^3}{3}\ \Bigr]_{-1}^2\\[5pt] |
| - | & =2+4-\frac{8}{3}-\frac{1}{2}+2-\frac{1}{3} \\ | + | &= \frac{2^2}{2} + 2\cdot 2 - \frac{2^3}{3} - \Bigl( \frac{(-1)^2}{2} + 2\cdot (-1) - \frac{(-1)^3}{3}\Bigr)\\[5pt] |
| - | & =\frac{9}{2} \\ | + | &= 2 + 4 - \frac{8}{3} - \frac{1}{2} + 2 - \frac{1}{3}\\[5pt] |
| - | \end{align}</math> | + | &= \frac{9}{2}\,\textrm{.} |
| + | \end{align}</math>}} | ||
Version vom 09:18, 28. Okt. 2008
The double inequality means that we look for the area of the region which is bounded above in the y-direction by the straight line \displaystyle y=x+2 and from below by the parabola \displaystyle y=x^2.
If we sketch the line and the parabola, the region is given by the region shaded in the figure below.
As soon as we have determined the x-coordinates of the points of intersection, \displaystyle x=a and \displaystyle x=b, between the line and the parabola, we can calculate the area as the integral of the difference between the curves' y-values,
| \displaystyle \text{Area} = \int\limits_a^b \bigl(x+2-x^2\bigr)\,dx\,\textrm{.} |
The curves' points of intersection are those points which lie on both curves, i.e. which satisfy both curves' equations
| \displaystyle \left\{\begin{align}
y &= x+2\,,\\[5pt] y &= x^2\,\textrm{.} \end{align} \right. |
By eliminating \displaystyle y, we obtain an equation for \displaystyle x,
| \displaystyle x^{2}=x+2\,\textrm{.} |
If we move all x-terms to the left-hand side,
| \displaystyle x^2-x=2\,, |
and complete the square, we obtain
| \displaystyle \begin{align}
\Bigl(x-\frac{1}{2}\Bigr)^2 - \Bigl(\frac{1}{2}\Bigr)^2 &= 2\\[5pt] \Bigl(x-\frac{1}{2}\Bigr)^2 &= \frac{9}{4}\,\textrm{.} \end{align} |
Taking the root then gives that \displaystyle x=\tfrac{1}{2}\pm \tfrac{3}{2}. In other words, \displaystyle x=-1 and \displaystyle x=2\,.
The area of the region is now given by
| \displaystyle \begin{align}
\text{Area} &= \int\limits_{-1}^2 \bigl(x+2-x^2\bigr)\,dx\\[5pt] &= \Bigl[\ \frac{x^2}{2} + 2x - \frac{x^3}{3}\ \Bigr]_{-1}^2\\[5pt] &= \frac{2^2}{2} + 2\cdot 2 - \frac{2^3}{3} - \Bigl( \frac{(-1)^2}{2} + 2\cdot (-1) - \frac{(-1)^3}{3}\Bigr)\\[5pt] &= 2 + 4 - \frac{8}{3} - \frac{1}{2} + 2 - \frac{1}{3}\\[5pt] &= \frac{9}{2}\,\textrm{.} \end{align} |