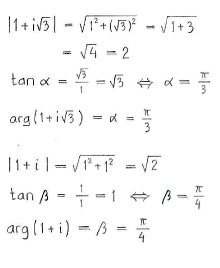Lösung 3.3:1d
Aus Online Mathematik Brückenkurs 2
K (Lösning 3.3:1d moved to Solution 3.3:1d: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
| - | { | + | Because we are going to raise something to the power |
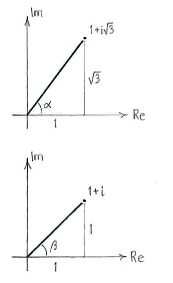
| - | < | + | <math>\text{12}</math>, the base in the expression should be written in polar form. In turn, the base consists of a quotient which it is advantageous to calculate in polar form. Thus, it seems appropriate to write |
| - | + | <math>1+i\sqrt{3}</math> | |
| - | { | + | and |
| - | < | + | <math>\text{1}+i</math> in polar form right from the beginning and to carry out all calculations in polar form. |
| - | + | ||
[[Image:3_3_1_d.gif]] [[Image:3_3_1_d_text.gif]] | [[Image:3_3_1_d.gif]] [[Image:3_3_1_d_text.gif]] | ||
| + | |||
| + | |||
| + | We obtain | ||
| + | |||
| + | |||
| + | <math>\begin{align} | ||
| + | & 1+i\sqrt{3}=2\left( \cos \frac{\pi }{3}+i\sin \frac{\pi }{3} \right) \\ | ||
| + | & \text{1}+i=\sqrt{2}\left( \cos \frac{\pi }{4}+i\sin \frac{\pi }{4} \right) \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | |||
| + | and | ||
| + | |||
| + | |||
| + | <math>\begin{align} | ||
| + | & \frac{1+i\sqrt{3}}{\text{1}+i}=\frac{2\left( \cos \frac{\pi }{3}+i\sin \frac{\pi }{3} \right)}{\sqrt{2}\left( \cos \frac{\pi }{4}+i\sin \frac{\pi }{4} \right)} \\ | ||
| + | & =\frac{2}{\sqrt{2}}\left( \cos \left( \frac{\pi }{3}-\frac{\pi }{4} \right)+i\sin \left( \frac{\pi }{3}-\frac{\pi }{4} \right) \right) \\ | ||
| + | & =\sqrt{2}\left( \cos \frac{\pi }{12}+i\sin \frac{\pi }{12} \right) \\ | ||
| + | & \\ | ||
| + | & \\ | ||
| + | & \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | |||
| + | Finally, de Moivre's formula gives | ||
| + | |||
| + | |||
| + | <math>\begin{align} | ||
| + | & \left( \frac{1+i\sqrt{3}}{\text{1}+i} \right)^{12}=\left( \sqrt{2} \right)^{12}\left( \cos 12\centerdot \frac{\pi }{12}+i\sin 12\centerdot \frac{\pi }{12} \right) \\ | ||
| + | & =2^{\frac{1}{2}\centerdot 12}\left( \cos \pi +i\sin \pi \right) \\ | ||
| + | & =2^{6}\centerdot \left( -1+i\centerdot 0 \right) \\ | ||
| + | & =-64 \\ | ||
| + | \end{align}</math> | ||
Version vom 07:49, 24. Okt. 2008
Because we are going to raise something to the power \displaystyle \text{12}, the base in the expression should be written in polar form. In turn, the base consists of a quotient which it is advantageous to calculate in polar form. Thus, it seems appropriate to write \displaystyle 1+i\sqrt{3} and \displaystyle \text{1}+i in polar form right from the beginning and to carry out all calculations in polar form.
We obtain
\displaystyle \begin{align}
& 1+i\sqrt{3}=2\left( \cos \frac{\pi }{3}+i\sin \frac{\pi }{3} \right) \\
& \text{1}+i=\sqrt{2}\left( \cos \frac{\pi }{4}+i\sin \frac{\pi }{4} \right) \\
\end{align}
and
\displaystyle \begin{align}
& \frac{1+i\sqrt{3}}{\text{1}+i}=\frac{2\left( \cos \frac{\pi }{3}+i\sin \frac{\pi }{3} \right)}{\sqrt{2}\left( \cos \frac{\pi }{4}+i\sin \frac{\pi }{4} \right)} \\
& =\frac{2}{\sqrt{2}}\left( \cos \left( \frac{\pi }{3}-\frac{\pi }{4} \right)+i\sin \left( \frac{\pi }{3}-\frac{\pi }{4} \right) \right) \\
& =\sqrt{2}\left( \cos \frac{\pi }{12}+i\sin \frac{\pi }{12} \right) \\
& \\
& \\
& \\
\end{align}
Finally, de Moivre's formula gives
\displaystyle \begin{align}
& \left( \frac{1+i\sqrt{3}}{\text{1}+i} \right)^{12}=\left( \sqrt{2} \right)^{12}\left( \cos 12\centerdot \frac{\pi }{12}+i\sin 12\centerdot \frac{\pi }{12} \right) \\
& =2^{\frac{1}{2}\centerdot 12}\left( \cos \pi +i\sin \pi \right) \\
& =2^{6}\centerdot \left( -1+i\centerdot 0 \right) \\
& =-64 \\
\end{align}