Lösung 3.2:5a

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Lösning 3.2:5a moved to Solution 3.2:5a: Robot: moved page)
Zeile 1: Zeile 1:
-
{{NAVCONTENT_START}}
+
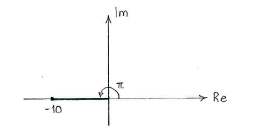
The argument of a complex number is angle of the line between the origin and the number measured with respect the positive real number axis.
-
<center> [[Image:3_2_5a.gif]] </center>
+
 
-
{{NAVCONTENT_STOP}}
+
In this case, we see directly that
 +
<math>-\text{1}0</math>
 +
has the argument
 +
<math>\pi </math>.
 +
 
[[Image:3_2_5_a.gif|center]]
[[Image:3_2_5_a.gif|center]]
 +
 +
 +
NOTE: All angles that correspond to the same direction differ by a whole number of turns, i.e. a multiple of
 +
<math>2\pi </math>, and therefore we could have just as well answered
 +
<math>-\pi </math>,
 +
<math>3\pi </math>,
 +
<math>5\pi </math>
 +
etc. However, it is usual to give the argument between
 +
<math>0</math>
 +
and
 +
<math>2\pi </math>
 +
or between
 +
<math>-\pi </math>
 +
and
 +
<math>\pi </math>.

Version vom 15:46, 22. Okt. 2008

The argument of a complex number is angle of the line between the origin and the number measured with respect the positive real number axis.

In this case, we see directly that \displaystyle -\text{1}0 has the argument \displaystyle \pi .



NOTE: All angles that correspond to the same direction differ by a whole number of turns, i.e. a multiple of \displaystyle 2\pi , and therefore we could have just as well answered \displaystyle -\pi , \displaystyle 3\pi , \displaystyle 5\pi etc. However, it is usual to give the argument between \displaystyle 0 and \displaystyle 2\pi or between \displaystyle -\pi and \displaystyle \pi .

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.2:5a“