Lösung 1.3:4

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
Zeile 1: Zeile 1:
-
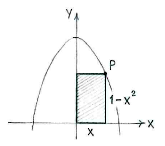
If we call the
+
If we call the ''x''-coordinate of the point <math>P</math> <math>x</math>, then its ''y''-coordinate is <math>1-x^{2}</math>, because <math>P</math> lies on the curve <math>y=1-x^{2}</math>.
-
<math>x</math>
+
-
-coordinate of the point
+
-
<math>P</math>
+
-
<math>x</math>, then its
+
[[Image:1_3_4-1-1.gif|center]]
-
<math>y</math>
+
-
-coordinate is
+
-
<math>1-x^{2}</math>, because
+
-
<math>P</math>
+
-
lies on the curve
+
-
<math>y=1-x^{2}</math>.
+
-
 
+
-
FIGURE
+
The area of the rectangle is then given by
The area of the rectangle is then given by
 +
{{Displayed math||<math>A(x) = \text{(base)}\cdot\text{(height)} = x\cdot (1-x^2)</math>}}
-
<math>A\left( x \right)=</math>
+
and we will try to choose <math>x</math> so that this area function is maximised.
-
base *height
+
-
<math>=x\centerdot \left( 1-x^{2} \right)</math>.
+
-
 
+
To begin with, we note that, because <math>P</math> should lie in the first quadrant, <math>x\ge 0</math> and also <math>y=1-x^2\ge 0</math>, i.e. <math>x\le 1</math>. We should therefore look for the maximum of <math>A(x)</math> when <math>0\le x\le 1\,</math>.
-
 
+
-
and we will try to choose
+
-
<math>x</math>
+
-
so that this area function is maximised.
+
-
 
+
-
To begin with, we note that, because
+
-
<math>P</math>
+
-
should lie in the first quadrant,
+
-
<math>x\ge 0</math>
+
-
and also
+
-
<math>y=1-x^{2}\ge 0</math>, i.e.
+
-
<math>x\le 1</math>. We should therefore look for the maximum of
+
-
<math>A\left( x \right)</math>
+
-
when
+
-
<math>0\le x\le 1</math>.
+
There are three types of points which can maximise the area function:
There are three types of points which can maximise the area function:
-
1. critical points,
+
# critical points,
-
2. points where the function is not differentiable,
+
# points where the function is not differentiable,
-
3. endpoints of the region of definition.
+
# endpoints of the region of definition.
-
 
+
-
The function
+
-
<math>A\left( x \right)=x\left( 1-x^{2} \right)</math>
+
-
is differentiable everywhere, so item 2. does not apply. In addition,
+
-
<math>A\left( 0 \right)=A\left( 1 \right)=0</math>, so the endpoints in item 3. cannot be maximum points (but rather the opposite, i.e. minimum points).
+
-
 
+
-
We must therefore supposed that the maximum area is a critical point. We differentiate
+
 +
The function <math>A(x) = x(1-x^2)</math> is differentiable everywhere, so item 2 does not apply. In addition, <math>A(0) = A(1) = 0\,</math>, so the endpoints in item 3 cannot be maximum points (but rather the opposite, i.e. minimum points).
-
<math>{A}'\left( x \right)=1\centerdot \left( 1-x^{2} \right)+x\centerdot \left( -2x \right)=1-3x^{2}</math>,
+
We must therefore conclude that the maximum area is a critical point. We differentiate
-
and the condition that the derivative should be zero gives that
+
{{Displayed math||<math>A'(x) = 1\cdot (1-x^2) + x\cdot (-2x) = 1-3x^2\,,</math>}}
-
<math>x=\pm {1}/{\sqrt{3}}\;</math>; however, it is only
+
-
<math>x={1}/{\sqrt{3}}\;</math>
+
-
which satisfies
+
-
<math>0\le x\le 1</math>
+
-
At the critical point, the second derivative
+
-
<math>{A}''</math>
+
-
has the value
+
 +
and the condition that the derivative should be zero gives that <math>x=\pm 1/\!\sqrt{3}</math>; however, it is only <math>x=1/\!\sqrt{3}</math> which satisfies <math>0\le x\le 1</math>.
-
<math>{A}''\left( {1}/{\sqrt{3}}\; \right)=-6\centerdot \frac{1}{\sqrt{3}}<0</math>,
+
At the critical point, the second derivative <math>A''(x)=-6x</math> has the value
-
which shows that
+
{{Displayed math||<math>A''\bigl( 1/\!\sqrt{3}\bigr) = -6\cdot\frac{1}{\sqrt{3}} < 0\,,</math>}}
-
<math>{1}/{\sqrt{3}}\;</math>
+
-
is a local maximum.
+
-
The answer is that the point
+
which shows that <math>x=1/\!\sqrt{3}</math> is a local maximum.
-
<math>P</math>
+
-
should be chosen so that
+
 +
The answer is that the point <math>P</math> should be chosen so that
-
<math>P=\left( \frac{1}{\sqrt{3}} \right.,\left. 1-\left( \frac{1}{\sqrt{3}} \right)^{2} \right)=\left( \frac{1}{\sqrt{3}} \right.,\left. \frac{2}{3} \right)</math>.
+
{{Displayed math||<math>P = \Bigl(\frac{1}{\sqrt{3}}, 1-\Bigl(\frac{1}{\sqrt{3}} \Bigr)^2\, \Bigr) = \Bigl(\frac{1}{\sqrt{3}}, \frac{2}{3} \Bigr)\,\textrm{.}</math>}}

Version vom 08:43, 21. Okt. 2008

If we call the x-coordinate of the point \displaystyle P \displaystyle x, then its y-coordinate is \displaystyle 1-x^{2}, because \displaystyle P lies on the curve \displaystyle y=1-x^{2}.

The area of the rectangle is then given by

\displaystyle A(x) = \text{(base)}\cdot\text{(height)} = x\cdot (1-x^2)

and we will try to choose \displaystyle x so that this area function is maximised.

To begin with, we note that, because \displaystyle P should lie in the first quadrant, \displaystyle x\ge 0 and also \displaystyle y=1-x^2\ge 0, i.e. \displaystyle x\le 1. We should therefore look for the maximum of \displaystyle A(x) when \displaystyle 0\le x\le 1\,.

There are three types of points which can maximise the area function:

  1. critical points,
  2. points where the function is not differentiable,
  3. endpoints of the region of definition.

The function \displaystyle A(x) = x(1-x^2) is differentiable everywhere, so item 2 does not apply. In addition, \displaystyle A(0) = A(1) = 0\,, so the endpoints in item 3 cannot be maximum points (but rather the opposite, i.e. minimum points).

We must therefore conclude that the maximum area is a critical point. We differentiate

\displaystyle A'(x) = 1\cdot (1-x^2) + x\cdot (-2x) = 1-3x^2\,,

and the condition that the derivative should be zero gives that \displaystyle x=\pm 1/\!\sqrt{3}; however, it is only \displaystyle x=1/\!\sqrt{3} which satisfies \displaystyle 0\le x\le 1.

At the critical point, the second derivative \displaystyle A''(x)=-6x has the value

\displaystyle A''\bigl( 1/\!\sqrt{3}\bigr) = -6\cdot\frac{1}{\sqrt{3}} < 0\,,

which shows that \displaystyle x=1/\!\sqrt{3} is a local maximum.

The answer is that the point \displaystyle P should be chosen so that

\displaystyle P = \Bigl(\frac{1}{\sqrt{3}}, 1-\Bigl(\frac{1}{\sqrt{3}} \Bigr)^2\, \Bigr) = \Bigl(\frac{1}{\sqrt{3}}, \frac{2}{3} \Bigr)\,\textrm{.}
Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_1.3:4“