Lösung 2.1:1a
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K (Lösning 2.1:1a moved to Solution 2.1:1a: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
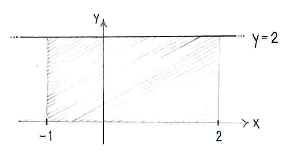
| - | + | The integral's value can be interpreted as the area under the graph | |
| - | < | + | <math>y=2</math> |
| - | { | + | from |
| + | <math>x=-1\ </math> | ||
| + | to | ||
| + | <math>\text{ }x=2</math>. | ||
| + | |||
[[Image:2_1_1_a.gif|center]] | [[Image:2_1_1_a.gif|center]] | ||
| + | |||
| + | Because the region is a rectangle, we can determine its area directly and obtain | ||
| + | |||
| + | |||
| + | <math>\int\limits_{-1}^{2}{2dx=}</math> | ||
| + | (base) | ||
| + | <math>\centerdot </math> | ||
| + | (height) | ||
| + | <math>=3\centerdot 2=6</math> | ||
Version vom 11:29, 17. Okt. 2008
The integral's value can be interpreted as the area under the graph \displaystyle y=2 from \displaystyle x=-1\ to \displaystyle \text{ }x=2.
Because the region is a rectangle, we can determine its area directly and obtain
\displaystyle \int\limits_{-1}^{2}{2dx=}
(base)
\displaystyle \centerdot
(height)
\displaystyle =3\centerdot 2=6