Lösung 3.2:2e
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
| Zeile 1: | Zeile 1: | ||
| - | Nachdem die Gleichung <math>z</math> und <math>\bar{z}</math> enthält, schreiben wir <math>z=x+iy</math>, | + | Nachdem die Gleichung <math>z</math> und <math>\bar{z}</math> enthält, schreiben wir <math>z=x+iy</math>, wobei <math>x</math> der Realteil von <math>z</math> ist und <math>y</math> der Imaginärteil ist. Wir erhalten also |
:*<math>\mathop{\rm Re}z = x</math> | :*<math>\mathop{\rm Re}z = x</math> | ||
:*<math>i+\bar{z} = i+(x-iy) = x+(1-y)i</math> | :*<math>i+\bar{z} = i+(x-iy) = x+(1-y)i</math> | ||
| - | Und unsere | + | Und unsere Gleichungen zeigen uns |
{{Abgesetzte Formel||<math>x=x+(1-y)i\quad\Leftrightarrow\quad 0=(1-y)i</math>}} | {{Abgesetzte Formel||<math>x=x+(1-y)i\quad\Leftrightarrow\quad 0=(1-y)i</math>}} | ||
| - | + | daher ist <math>y=1</math>. | |
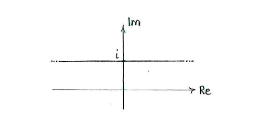
Also besteht unsere Fläche aus allen komlexen Zahlen deren Imaginärteil 1 ist. | Also besteht unsere Fläche aus allen komlexen Zahlen deren Imaginärteil 1 ist. | ||
[[Image:3_2_2_e.gif|center]] | [[Image:3_2_2_e.gif|center]] | ||
Version vom 16:08, 22. Aug. 2009
Nachdem die Gleichung \displaystyle z und \displaystyle \bar{z} enthält, schreiben wir \displaystyle z=x+iy, wobei \displaystyle x der Realteil von \displaystyle z ist und \displaystyle y der Imaginärteil ist. Wir erhalten also
- \displaystyle \mathop{\rm Re}z = x
- \displaystyle i+\bar{z} = i+(x-iy) = x+(1-y)i
Und unsere Gleichungen zeigen uns
| \displaystyle x=x+(1-y)i\quad\Leftrightarrow\quad 0=(1-y)i |
daher ist \displaystyle y=1.
Also besteht unsere Fläche aus allen komlexen Zahlen deren Imaginärteil 1 ist.