Lösung 3.2:6a

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Solution 3.2:6a moved to Lösung 3.2:6a: Robot: moved page)
Zeile 1: Zeile 1:
-
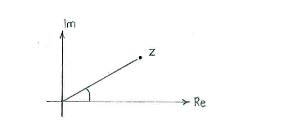
Polar form is an alternative way to express complex numbers. The idea is that it is sufficient to know a complex number's distance from the origin (magnitude) and the angle which the line between the number and the origin makes with the positive real axis (argument), in order to determine the number.
+
Die Polarform ist nur eine Art komplexe Zahlen zu schreiben. Nachdem ein Vektor eindeutig durch seinen Betrag und Argument (Winkel) definiert ist, gilt dasselbe auch für komplexe Zahlen.
[[Image:3_2_6_a.gif|center]]
[[Image:3_2_6_a.gif|center]]
-
Instead of working with the number's rectangular form,
+
Anstatt dass wir die komplexe Zahl wie
-
<math>x+iy</math>, we use its magnitude and argument to obtain a simpler and more natural description of e.g. multiplication and division.
+
<math>x+iy</math> schreiben, schreiben wir sie in Polarform, um unter anderen Multiplikation und Division zu vereinfachen.
-
The relation between a complex number in rectangular form and in polar form can be written as
+
Die Polarform wird definiert durch:
{{Abgesetzte Formel||<math>x+iy=r(\cos\alpha + i\sin\alpha)\,,</math>}}
{{Abgesetzte Formel||<math>x+iy=r(\cos\alpha + i\sin\alpha)\,,</math>}}
-
where <math>x</math> and <math>y</math> are the number's real and imaginary parts respectively, and where <math>r</math> and <math>\alpha</math> are the magnitude and the argument, respectively. It is the right-hand side in this formula which is usually said to be the number's polar form.
+
wo <math>x</math> und <math>y</math> reelle Zahlen sind, und <math>r</math> und <math>\alpha</math> der Betrag und das Argument sind. Die rechte Seite der Formen benennt man die Polarform einer komplexen Zahl.
-
The number <math>\text{3}</math> has magnitude <math>\text{3}</math> (distance to the origin), and argument <math>0</math> because the number lies on the positive real axis. The number's polar form is therefore
+
Die Zahl 3 hat den Betrag 3, und das Argument <math>0</math>, nachdem sie auf der reellen Achse liegt. Daher ist
{{Abgesetzte Formel||<math>3(\cos 0 + i\sin 0)\,\textrm{.}</math>}}
{{Abgesetzte Formel||<math>3(\cos 0 + i\sin 0)\,\textrm{.}</math>}}

Version vom 13:04, 13. Mai 2009

Die Polarform ist nur eine Art komplexe Zahlen zu schreiben. Nachdem ein Vektor eindeutig durch seinen Betrag und Argument (Winkel) definiert ist, gilt dasselbe auch für komplexe Zahlen.

Anstatt dass wir die komplexe Zahl wie \displaystyle x+iy schreiben, schreiben wir sie in Polarform, um unter anderen Multiplikation und Division zu vereinfachen.

Die Polarform wird definiert durch:

\displaystyle x+iy=r(\cos\alpha + i\sin\alpha)\,,

wo \displaystyle x und \displaystyle y reelle Zahlen sind, und \displaystyle r und \displaystyle \alpha der Betrag und das Argument sind. Die rechte Seite der Formen benennt man die Polarform einer komplexen Zahl.

Die Zahl 3 hat den Betrag 3, und das Argument \displaystyle 0, nachdem sie auf der reellen Achse liegt. Daher ist

\displaystyle 3(\cos 0 + i\sin 0)\,\textrm{.}
Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.2:6a“