Lösung 2.1:4a

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Solution 2.1:4a moved to Lösung 2.1:4a: Robot: moved page)
Zeile 1: Zeile 1:
-
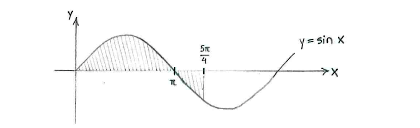
If we draw the curve <math>y=\sin x</math>, we see that the curve lies above the ''x''-axis as far as <math>x=\pi </math> and then lies under the ''x''-axis.
+
Zeichnen wir die Funktion <math>y=\sin x</math>, sehen wir dass die Funktion bis <math>x=\pi </math> oberhalb der ''x''-Achse liegt, und danach unterhalb.
[[Image:2_1_4_a1.gif|center]]
[[Image:2_1_4_a1.gif|center]]
-
The area of the region between <math>x=0</math> and <math>x=\pi</math> can therefore be written as
+
Die Fläche vom Gebiet zwischen <math>x=0</math> und <math>x=\pi</math> ist deshalb
{{Abgesetzte Formel||<math>\int\limits_{0}^{\pi} \sin x\,dx</math>}}
{{Abgesetzte Formel||<math>\int\limits_{0}^{\pi} \sin x\,dx</math>}}
-
whilst the area of the remaining region under the ''x''-axis is equal to
+
während die Fläche vom restierenden Gebiet
{{Abgesetzte Formel||<math>-\int\limits_{\pi}^{5\pi/4} \sin x\,dx</math>}}
{{Abgesetzte Formel||<math>-\int\limits_{\pi}^{5\pi/4} \sin x\,dx</math>}}
-
(note the minus sign in front of the integral).
+
ist (beachten Sie das Minuszeichen).
-
The total area becomes
+
Die gesamte Fläche ist also
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}
Zeile 24: Zeile 24:
\end{align}</math>}}
\end{align}</math>}}
-
 
+
Hinweis: Die exakten Werte von <math>\cos 0</math>, <math>\cos \pi </math> und <math>\cos (5\pi/4)</math> können wir durch den Einheitskreis erhalten, indem wir die Winkeln <math>0</math>, <math>\pi</math> und <math>5\pi/4</math> einzeichnen, und deren ''x''-Koordinaten ablesen.
-
Note: A simple way to obtain the values of <math>\cos 0</math>, <math>\cos \pi </math> and <math>\cos (5\pi/4)</math> is to draw the angles <math>0</math>, <math>\pi</math> and <math>5\pi/4</math> on a unit circle and to read off the cosine value as the ''x''-coordinate for the corresponding point on the circle.
+
[[Image:2_1_4_a2.gif|center]]
[[Image:2_1_4_a2.gif|center]]

Version vom 17:02, 28. Apr. 2009

Zeichnen wir die Funktion \displaystyle y=\sin x, sehen wir dass die Funktion bis \displaystyle x=\pi oberhalb der x-Achse liegt, und danach unterhalb.

Die Fläche vom Gebiet zwischen \displaystyle x=0 und \displaystyle x=\pi ist deshalb

\displaystyle \int\limits_{0}^{\pi} \sin x\,dx

während die Fläche vom restierenden Gebiet

\displaystyle -\int\limits_{\pi}^{5\pi/4} \sin x\,dx

ist (beachten Sie das Minuszeichen).

Die gesamte Fläche ist also

\displaystyle \begin{align}

& \int\limits_{0}^{\pi} \sin x\,dx - \int\limits_{\pi}^{5\pi/4} \sin x\,dx\\[5pt] &\qquad\quad {}= \Bigl[\ -\cos x\ \Bigr]_0^\pi - \Bigl[\ -\cos x\ \Bigr]_\pi^{5\pi/4}\\[5pt] &\qquad\quad {}= \Bigl( -\cos\pi - (-\cos 0)\Bigr) - \Bigl( -\cos\frac{5\pi}{4} - (-\cos\pi) \Bigr)\\[5pt] &\qquad\quad {}= \Bigl( -(-1)-(-1) \Bigr) - \Bigl( -\Bigl(-\frac{1}{\sqrt{2}} \Bigr) - \bigl(-(-1)\bigr)\Bigr)\\[5pt] &\qquad\quad {}= 1+1-\frac{1}{\sqrt{2}}+1\\[5pt] &\qquad\quad {}= 3-\frac{1}{\sqrt{2}}\,\textrm{.} \end{align}

Hinweis: Die exakten Werte von \displaystyle \cos 0, \displaystyle \cos \pi und \displaystyle \cos (5\pi/4) können wir durch den Einheitskreis erhalten, indem wir die Winkeln \displaystyle 0, \displaystyle \pi und \displaystyle 5\pi/4 einzeichnen, und deren x-Koordinaten ablesen.

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_2.1:4a“