Lösung 3.3:2a
Aus Online Mathematik Brückenkurs 2
K |
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
||
| Zeile 3: | Zeile 3: | ||
We start by writing <math>z</math> and <math>1</math> in polar form | We start by writing <math>z</math> and <math>1</math> in polar form | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align} |
z &= r(\cos\alpha + i\sin\alpha)\,,\\[5pt] | z &= r(\cos\alpha + i\sin\alpha)\,,\\[5pt] | ||
1 &= 1(\cos 0 + i\sin 0)\,\textrm{.} | 1 &= 1(\cos 0 + i\sin 0)\,\textrm{.} | ||
| Zeile 10: | Zeile 10: | ||
The equation then becomes | The equation then becomes | ||
| - | {{ | + | {{Abgesetzte Formel||<math>r^4(\cos 4\alpha + i\sin 4\alpha) = 1\,(\cos 0 + i\sin 0)\,,</math>}} |
where we have used de Moivre's formula on the left-hand side. In order that both sides are equal, they must have the same magnitude and the same argument to within a multiple of <math>2\pi</math>, i.e. | where we have used de Moivre's formula on the left-hand side. In order that both sides are equal, they must have the same magnitude and the same argument to within a multiple of <math>2\pi</math>, i.e. | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\left\{\begin{align} |
r^{4} &= 1\,,\\[5pt] | r^{4} &= 1\,,\\[5pt] | ||
4\alpha &= 0+2n\pi\,,\quad (\text{n is an arbitrary integer})\,\textrm{.} | 4\alpha &= 0+2n\pi\,,\quad (\text{n is an arbitrary integer})\,\textrm{.} | ||
| Zeile 21: | Zeile 21: | ||
This means that | This means that | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\left\{\begin{align} |
r &= 1\,,\\[5pt] | r &= 1\,,\\[5pt] | ||
\alpha &= \frac{n\pi}{2}\,,\quad \text{(n is an arbitrary integer).} | \alpha &= \frac{n\pi}{2}\,,\quad \text{(n is an arbitrary integer).} | ||
| Zeile 28: | Zeile 28: | ||
The solutions are thus (in polar form) | The solutions are thus (in polar form) | ||
| - | {{ | + | {{Abgesetzte Formel||<math>z = 1\cdot\Bigl(\cos\frac{n\pi}{2} + i\sin\frac{n\pi}{2}\Bigr)\,,\quad\text{for }n=0,\ \pm 1,\ \pm 2,\ldots</math>}} |
but observe that the argument on the right-hand side essentially takes only four different values <math>0</math>, <math>\pi/2</math>, <math>\pi</math> and <math>3\pi/2\,</math>, because other values of <math>n</math> give some of these values plus/minus a multiple of <math>2\pi\,</math>. | but observe that the argument on the right-hand side essentially takes only four different values <math>0</math>, <math>\pi/2</math>, <math>\pi</math> and <math>3\pi/2\,</math>, because other values of <math>n</math> give some of these values plus/minus a multiple of <math>2\pi\,</math>. | ||
| Zeile 34: | Zeile 34: | ||
The equation's solutions are therefore | The equation's solutions are therefore | ||
| - | {{ | + | {{Abgesetzte Formel||<math>z=\left\{\begin{align} |
&1\cdot(\cos 0 + i\sin 0)\,,\\[5pt] | &1\cdot(\cos 0 + i\sin 0)\,,\\[5pt] | ||
&1\cdot(\cos (\pi/2) + i\sin (\pi/2))\,,\\[5pt] | &1\cdot(\cos (\pi/2) + i\sin (\pi/2))\,,\\[5pt] | ||
Version vom 13:11, 10. Mär. 2009
An equation of the type "\displaystyle z^{n} = \text{a complex number}" is called a binomial equation and these are usually solved by going over to polar form and using de Moivre's formula.
We start by writing \displaystyle z and \displaystyle 1 in polar form
| \displaystyle \begin{align}
z &= r(\cos\alpha + i\sin\alpha)\,,\\[5pt] 1 &= 1(\cos 0 + i\sin 0)\,\textrm{.} \end{align} |
The equation then becomes
| \displaystyle r^4(\cos 4\alpha + i\sin 4\alpha) = 1\,(\cos 0 + i\sin 0)\,, |
where we have used de Moivre's formula on the left-hand side. In order that both sides are equal, they must have the same magnitude and the same argument to within a multiple of \displaystyle 2\pi, i.e.
| \displaystyle \left\{\begin{align}
r^{4} &= 1\,,\\[5pt] 4\alpha &= 0+2n\pi\,,\quad (\text{n is an arbitrary integer})\,\textrm{.} \end{align}\right. |
This means that
| \displaystyle \left\{\begin{align}
r &= 1\,,\\[5pt] \alpha &= \frac{n\pi}{2}\,,\quad \text{(n is an arbitrary integer).} \end{align}\right. |
The solutions are thus (in polar form)
| \displaystyle z = 1\cdot\Bigl(\cos\frac{n\pi}{2} + i\sin\frac{n\pi}{2}\Bigr)\,,\quad\text{for }n=0,\ \pm 1,\ \pm 2,\ldots |
but observe that the argument on the right-hand side essentially takes only four different values \displaystyle 0, \displaystyle \pi/2, \displaystyle \pi and \displaystyle 3\pi/2\,, because other values of \displaystyle n give some of these values plus/minus a multiple of \displaystyle 2\pi\,.
The equation's solutions are therefore
| \displaystyle z=\left\{\begin{align}
&1\cdot(\cos 0 + i\sin 0)\,,\\[5pt] &1\cdot(\cos (\pi/2) + i\sin (\pi/2))\,,\\[5pt] &1\cdot(\cos \pi + i\sin \pi)\,,\\[5pt] &1\cdot(\cos (3\pi/2) + i\sin (3\pi/2))\,, \end{align}\right. = \left\{ \begin{align} 1\,,&\\[5pt] i\,,&\\[5pt] -1\,,&\\[5pt] -i\,\textrm{.}& \end{align}\right. |
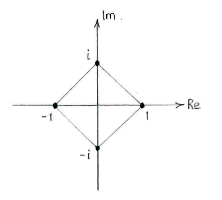
Note: If we mark these solutions on the complex number plane, we see that they are corners in a regular quadrilateral.