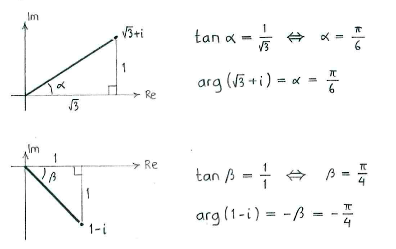Lösung 3.2:5c

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel))
Zeile 1: Zeile 1:
Geometrically, the multiplication of two complex numbers means that there magnitudes are multiplied and their arguments are added. The product <math>(\sqrt{3}+i)(1-i)</math> therefore has an argument which is the sum of the argument for the <math>\sqrt{3}+i</math> and <math>1-i</math>, i.e.
Geometrically, the multiplication of two complex numbers means that there magnitudes are multiplied and their arguments are added. The product <math>(\sqrt{3}+i)(1-i)</math> therefore has an argument which is the sum of the argument for the <math>\sqrt{3}+i</math> and <math>1-i</math>, i.e.
-
{{Displayed math||<math>\arg \bigl((\sqrt{3}+i)(1-i)\bigr) = \arg (\sqrt{3}+i) + \arg (1-i)\,\textrm{.}</math>}}
+
{{Abgesetzte Formel||<math>\arg \bigl((\sqrt{3}+i)(1-i)\bigr) = \arg (\sqrt{3}+i) + \arg (1-i)\,\textrm{.}</math>}}
By drawing the factors in the complex plane, we can determine relatively easily the argument using simple trigonometry.
By drawing the factors in the complex plane, we can determine relatively easily the argument using simple trigonometry.
Zeile 12: Zeile 12:
Hence,
Hence,
-
{{Displayed math||<math>\arg \bigl((\sqrt{3}+i)(1-i)\bigr) = \arg (\sqrt{3}+i) + \arg (1-i) = \frac{\pi}{6} - \frac{\pi}{4} = -\frac{\pi}{12}\,\textrm{.}</math>}}
+
{{Abgesetzte Formel||<math>\arg \bigl((\sqrt{3}+i)(1-i)\bigr) = \arg (\sqrt{3}+i) + \arg (1-i) = \frac{\pi}{6} - \frac{\pi}{4} = -\frac{\pi}{12}\,\textrm{.}</math>}}
Note: If you prefer to give the argument between <math>0</math> and <math>2\pi </math>, then the answer is
Note: If you prefer to give the argument between <math>0</math> and <math>2\pi </math>, then the answer is
-
{{Displayed math||<math>-\frac{\pi}{12}+2\pi = \frac{-\pi+24\pi}{12} = \frac{23\pi}{12}\,\textrm{.}</math>}}
+
{{Abgesetzte Formel||<math>-\frac{\pi}{12}+2\pi = \frac{-\pi+24\pi}{12} = \frac{23\pi}{12}\,\textrm{.}</math>}}

Version vom 13:09, 10. Mär. 2009

Geometrically, the multiplication of two complex numbers means that there magnitudes are multiplied and their arguments are added. The product \displaystyle (\sqrt{3}+i)(1-i) therefore has an argument which is the sum of the argument for the \displaystyle \sqrt{3}+i and \displaystyle 1-i, i.e.

\displaystyle \arg \bigl((\sqrt{3}+i)(1-i)\bigr) = \arg (\sqrt{3}+i) + \arg (1-i)\,\textrm{.}

By drawing the factors in the complex plane, we can determine relatively easily the argument using simple trigonometry.

(Because \displaystyle 1-i lies in the fourth quadrant, the argument equals \displaystyle -\beta and not \displaystyle \beta.)

Hence,

\displaystyle \arg \bigl((\sqrt{3}+i)(1-i)\bigr) = \arg (\sqrt{3}+i) + \arg (1-i) = \frac{\pi}{6} - \frac{\pi}{4} = -\frac{\pi}{12}\,\textrm{.}


Note: If you prefer to give the argument between \displaystyle 0 and \displaystyle 2\pi , then the answer is

\displaystyle -\frac{\pi}{12}+2\pi = \frac{-\pi+24\pi}{12} = \frac{23\pi}{12}\,\textrm{.}
Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.2:5c“