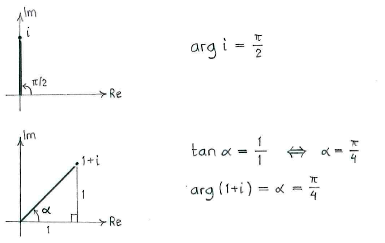Lösung 3.2:5d
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K |
|||
| Zeile 1: | Zeile 1: | ||
| - | When dividing two complex numbers, the numerator's magnitude is divided by the denominator's | + | When dividing two complex numbers, the numerator's magnitude is divided by the denominator's magnitude and the numerator's argument is subtracted from the numerator's argument. |
| - | The argument of the quotient | + | The argument of the quotient <math>i/(1+i)</math> is therefore |
| - | <math> | + | |
| - | is therefore | + | |
| + | {{Displayed math||<math>\arg\frac{i}{1+i} = \arg i - \arg (1+i)\,\textrm{.}</math>}} | ||
| - | + | We obtain the argument of <math>i</math> and <math>1+i</math> by drawing the numbers in the complex plane and using a little trigonometry. | |
| - | + | ||
| - | + | ||
| - | We obtain the argument of | + | |
| - | <math>i</math> | + | |
| - | and | + | |
| - | <math> | + | |
| - | by drawing the numbers in the complex plane and using a little trigonometry | + | |
| - | + | ||
[[Image:3_2_5_d.gif|center]] | [[Image:3_2_5_d.gif|center]] | ||
| - | |||
Hence, we obtain | Hence, we obtain | ||
| - | + | {{Displayed math||<math>\arg\frac{i}{1+i} = \arg i - \arg (1+i) = \frac{\pi}{2} - \frac{\pi}{4} = \frac{\pi}{4}\,\textrm{.}</math>}} | |
| - | <math>\arg \frac{i}{1+i}=\arg i-\arg | + | |
Version vom 12:43, 29. Okt. 2008
When dividing two complex numbers, the numerator's magnitude is divided by the denominator's magnitude and the numerator's argument is subtracted from the numerator's argument.
The argument of the quotient \displaystyle i/(1+i) is therefore
| \displaystyle \arg\frac{i}{1+i} = \arg i - \arg (1+i)\,\textrm{.} |
We obtain the argument of \displaystyle i and \displaystyle 1+i by drawing the numbers in the complex plane and using a little trigonometry.
Hence, we obtain
| \displaystyle \arg\frac{i}{1+i} = \arg i - \arg (1+i) = \frac{\pi}{2} - \frac{\pi}{4} = \frac{\pi}{4}\,\textrm{.} |