Lösung 3.3:2c
Aus Online Mathematik Brückenkurs 2
K (Lösning 3.3:2c moved to Solution 3.3:2c: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
| - | {{ | + | We write |
| - | < | + | <math>z\text{ }</math> |
| - | {{ | + | and the right-hand side |
| - | {{ | + | <math>\text{-1-}i</math> |
| - | < | + | in polar form |
| - | {{ | + | |
| + | |||
| + | <math>\begin{align} | ||
| + | & z=r\left( \cos \alpha +i\sin \alpha \right) \\ | ||
| + | & \text{-1-}i=\sqrt{2}\left( \cos \frac{5\pi }{4}+i\sin \frac{5\pi }{4} \right) \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | |||
| + | Using de Moivre's formula, the equation can now be written as | ||
| + | |||
| + | |||
| + | <math>r^{5}\left( \cos 5\alpha +i\sin 5\alpha \right)=\sqrt{2}\left( \cos \frac{5\pi }{4}+i\sin \frac{5\pi }{4} \right)</math> | ||
| + | |||
| + | |||
| + | If we identify the magnitude and argument on both sides, we get | ||
| + | |||
| + | |||
| + | <math>\left\{ \begin{array}{*{35}l} | ||
| + | r^{5}=\sqrt{2} \\ | ||
| + | 5\alpha =\frac{5\pi }{4}+2n\pi \quad \left( n\text{ an arbitrary integer} \right)\text{ } \\ | ||
| + | \end{array} \right.</math> | ||
| + | |||
| + | |||
| + | (The arguments | ||
| + | <math>5\alpha </math> | ||
| + | and | ||
| + | <math>\frac{5\pi }{4}</math> | ||
| + | can differ by a multiple of | ||
| + | <math>2\pi </math> | ||
| + | and still correspond to the same complex number.) | ||
| + | |||
| + | This gives that | ||
| + | |||
| + | |||
| + | <math>\left\{ \begin{array}{*{35}l} | ||
| + | r=\sqrt[5]{2}=\left( 2^{{1}/{2}\;} \right)^{{1}/{5}\;}=2^{{1}/{10}\;} \\ | ||
| + | \alpha =\frac{1}{5}\left( \frac{5\pi }{4}+2n\pi \right)=\frac{\pi }{4}+\frac{2n\pi }{5}\quad \left( n\text{ an arbitrary integer} \right)\text{ } \\ | ||
| + | \end{array} \right.</math> | ||
| + | |||
| + | |||
| + | If we investigate the argument | ||
| + | <math>\alpha </math> | ||
| + | more closely, we see that it assumes essentially only five different values, | ||
| + | |||
| + | |||
| + | <math>\frac{\pi }{4},\ \frac{\pi }{4}+\frac{2\pi }{5},\ \frac{\pi }{4}+\frac{4\pi }{5},\ \frac{\pi }{4}+\frac{6\pi }{5}</math> | ||
| + | and | ||
| + | <math>\ \frac{\pi }{4}+\frac{8\pi }{5}</math> | ||
| + | |||
| + | |||
| + | since these angle values then repeat to within a multiple of | ||
| + | <math>2\pi </math>. | ||
| + | |||
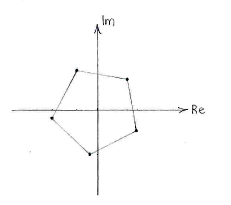
| + | In summary, the roots of the equation are | ||
| + | |||
| + | |||
| + | <math>z=2^{{1}/{10}\;}\left( \cos \left( \frac{\pi }{4}+\frac{2n\pi }{5} \right)+i\sin \left( \frac{\pi }{4}+\frac{2n\pi }{5} \right) \right)</math> | ||
| + | |||
| + | |||
| + | for | ||
| + | <math>n=0,\ 1,\ 2,\ 3</math> | ||
| + | and | ||
| + | <math>4</math> | ||
| + | |||
| + | |||
| + | |||
[[Image:3_3_2_c.gif|center]] | [[Image:3_3_2_c.gif|center]] | ||
Version vom 10:27, 24. Okt. 2008
We write \displaystyle z\text{ } and the right-hand side \displaystyle \text{-1-}i in polar form
\displaystyle \begin{align}
& z=r\left( \cos \alpha +i\sin \alpha \right) \\
& \text{-1-}i=\sqrt{2}\left( \cos \frac{5\pi }{4}+i\sin \frac{5\pi }{4} \right) \\
\end{align}
Using de Moivre's formula, the equation can now be written as
\displaystyle r^{5}\left( \cos 5\alpha +i\sin 5\alpha \right)=\sqrt{2}\left( \cos \frac{5\pi }{4}+i\sin \frac{5\pi }{4} \right)
If we identify the magnitude and argument on both sides, we get
\displaystyle \left\{ \begin{array}{*{35}l}
r^{5}=\sqrt{2} \\
5\alpha =\frac{5\pi }{4}+2n\pi \quad \left( n\text{ an arbitrary integer} \right)\text{ } \\
\end{array} \right.
(The arguments
\displaystyle 5\alpha
and
\displaystyle \frac{5\pi }{4}
can differ by a multiple of
\displaystyle 2\pi
and still correspond to the same complex number.)
This gives that
\displaystyle \left\{ \begin{array}{*{35}l}
r=\sqrt[5]{2}=\left( 2^{{1}/{2}\;} \right)^{{1}/{5}\;}=2^{{1}/{10}\;} \\
\alpha =\frac{1}{5}\left( \frac{5\pi }{4}+2n\pi \right)=\frac{\pi }{4}+\frac{2n\pi }{5}\quad \left( n\text{ an arbitrary integer} \right)\text{ } \\
\end{array} \right.
If we investigate the argument
\displaystyle \alpha
more closely, we see that it assumes essentially only five different values,
\displaystyle \frac{\pi }{4},\ \frac{\pi }{4}+\frac{2\pi }{5},\ \frac{\pi }{4}+\frac{4\pi }{5},\ \frac{\pi }{4}+\frac{6\pi }{5}
and
\displaystyle \ \frac{\pi }{4}+\frac{8\pi }{5}
since these angle values then repeat to within a multiple of
\displaystyle 2\pi .
In summary, the roots of the equation are
\displaystyle z=2^{{1}/{10}\;}\left( \cos \left( \frac{\pi }{4}+\frac{2n\pi }{5} \right)+i\sin \left( \frac{\pi }{4}+\frac{2n\pi }{5} \right) \right)
for
\displaystyle n=0,\ 1,\ 2,\ 3
and
\displaystyle 4