Lösung 1.3:5

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Solution 1.3:5 moved to Lösung 1.3:5: Robot: moved page)
Aktuelle Version (18:03, 9. Sep. 2009) (bearbeiten) (rückgängig)
 
(Der Versionsvergleich bezieht 4 dazwischen liegende Versionen mit ein.)
Zeile 1: Zeile 1:
-
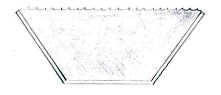
The channel holds most water when its cross-sectional area is greatest.
+
Der Kanal kann am meisten Wasser enthalten, wenn seine Querschnittsfläche am größten ist.
[[Image:1_3_5_1_1.gif|center]]
[[Image:1_3_5_1_1.gif|center]]
-
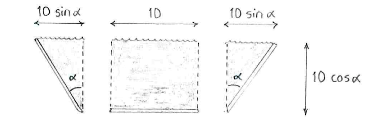
By dividing up the channel's cross-section into a rectangle and two triangles we can, with the help of a little trigonometry, determine the lengths of the rectangle's and triangles' sides, and thence the cross-sectional area.
+
Indem wir den Querschnitt des Kanals in ein Rechteck und zwei Dreiecke aufteilen, können wir mit ein wenig Trigonometrie die Querschnittsfläche des Kanals berechnen.
[[Image:1_3_5_1_2.gif|center]]
[[Image:1_3_5_1_2.gif|center]]
-
The area of the cross-section is
+
Die Fläche ist
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}
Zeile 14: Zeile 14:
\end{align}</math>}}
\end{align}</math>}}
-
If we limit the angle to lie between <math>0</math> and <math>\pi/2</math>, the problem can be formulated as:
+
Begrenzen wir den Winkel so, dass er zwischen <math>0</math> und <math>\pi/2</math> liegt, erhalten wir die folgende Optimierungsaufgabe:
-
::Maximise the function <math>A(\alpha) = 100\cos\alpha (1+\sin\alpha)</math> when
+
::Maximiere <math>A(\alpha) = 100\cos\alpha (1+\sin\alpha)</math> wenn <math>0\le \alpha \le {\pi }/{2}\,</math>.
-
<math>0\le \alpha \le {\pi }/{2}\,</math>.
+
-
The area function is a differentiable function and the area is least when <math>\alpha=0</math> or <math>\alpha=\pi/2\,</math>, so the area must assume its maximum at a critical point of the area function.
+
Die Funktion A ist überall differenzierbar und die Fläche ist minimal wenn <math>\alpha=0</math> oder wenn <math>\alpha=\pi/2\ </math>, also nimmt die Funktion ihr Maximum in einem stationären Punkt an.
-
We differentiate the area function:
+
Die Ableitung ist
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}
Zeile 28: Zeile 27:
\end{align}</math>}}
\end{align}</math>}}
-
At a critical point <math>A'(\alpha)=0</math> and this gives us the equation
+
Für stationäre Punkte ist <math>A'(\alpha)=0\,</math>. Das ergibt die Gleichung
-
{{Abgesetzte Formel||<math>\sin\alpha + \sin^2\!\alpha - \cos^2\!\alpha = 0</math>}}
+
{{Abgesetzte Formel||<math>\sin\alpha + \sin^2\!\alpha - \cos^2\!\alpha = 0\,.</math>}}
-
after eliminating the factor -100. We replace <math>\cos^2\!\alpha</math> with <math>1-\sin^2\!\alpha</math> (according to the Pythagorean identity) to obtain an equation solely in <math>\sin\alpha\,</math>,
+
Nachdem wir den Faktor -100 heraus gezogen haben, ersetzen wir <math>\cos^2\!\alpha</math> mit <math>1-\sin^2\!\alpha</math> und erhalten eine Gleichung mit nur <math>\sin\alpha\,</math>-Termen.
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}
\sin\alpha + \sin^2\!\alpha - (1-\sin^2\!\alpha) &= 0\\[5pt]
\sin\alpha + \sin^2\!\alpha - (1-\sin^2\!\alpha) &= 0\\[5pt]
-
2\sin^2\!\alpha + \sin\alpha - 1 &= 0\,\textrm{.}
+
2\sin^2\!\alpha + \sin\alpha - 1 &= 0\,\textrm{}
\end{align}</math>}}
\end{align}</math>}}
-
This is a second-degree equation in <math>\sin\alpha</math> and completing the square gives that
+
Das ist eine quadratische Gleichung für <math>\sin\alpha</math>. Durch quadratische Ergänzung erhalten wir
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}
2\bigl(\sin\alpha + \tfrac{1}{4}\bigr)^{2} - 2\bigl(\tfrac{1}{4}\bigr)^2 - 1 &= 0\\[5pt]
2\bigl(\sin\alpha + \tfrac{1}{4}\bigr)^{2} - 2\bigl(\tfrac{1}{4}\bigr)^2 - 1 &= 0\\[5pt]
\bigl(\sin\alpha + \tfrac{1}{4}\bigr)^2 &= \frac{9}{16}
\bigl(\sin\alpha + \tfrac{1}{4}\bigr)^2 &= \frac{9}{16}
-
\end{align}</math>}}
+
\end{align}</math>.}}
-
and we obtain <math>\sin\alpha = -\tfrac{1}{4}\pm \tfrac{3}{4}</math>, i.e. <math>\sin \alpha = -1</math> or <math>\sin \alpha = \tfrac{1}{2}\,</math>.
+
Wir erhalten weiter <math>\sin\alpha = -\tfrac{1}{4}\pm \tfrac{3}{4}</math>, also <math>\sin \alpha = -1</math> oder <math>\sin \alpha = \tfrac{1}{2}\,</math>.
-
The case <math>\sin \alpha =-1</math> is not satisfied for <math>0\le \alpha \le \pi/2</math> and <math>\sin \alpha = \tfrac{1}{2}</math> gives <math>\alpha = \pi/6</math>. Thus <math>\alpha = \pi/6\,</math> is a critical point.
+
Der Fall, dass <math>\sin \alpha =-1</math>, ist nie erfüllt für <math>0\le \alpha \le \pi/2</math> und <math>\sin \alpha = \tfrac{1}{2}</math> gibt <math>\alpha = \pi/6</math>. Also ist <math>\alpha = \pi/6\,</math> ein stationärer Punkt.
-
If we summarize, we know therefore that the cross-sectional area has local minimum points at <math>\alpha = 0</math> and <math>\alpha = \pi/2</math> and that we have a critical point at <math>\alpha = \pi/6\,</math>. This critical point must be a maximum, which we can also show using the second derivative,
+
Wir wissen von der Zeichnung her, dass die Fläche lokale Minima an den Stellen <math>\alpha = 0</math> und <math>\alpha = \pi/2</math> hat und die kritische Stelle <math>\alpha = \pi/6\,</math>. Daher muss die Funktion an der kritischen Stelle ein Maximum haben, wir zeigen dies mit der zweiten Ableitung.
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}
A''(\alpha) &= -100\cos\alpha - 100\cdot 2\sin\alpha\cdot\cos\alpha + 100\cdot 2\cos\alpha \cdot (-\sin\alpha)\\[5pt]
A''(\alpha) &= -100\cos\alpha - 100\cdot 2\sin\alpha\cdot\cos\alpha + 100\cdot 2\cos\alpha \cdot (-\sin\alpha)\\[5pt]
-
&= -100\cos\alpha (1+4\sin\alpha)\,,
+
&= -100\cos\alpha (1+4\sin\alpha)\,.
\end{align}</math>}}
\end{align}</math>}}
-
which is negative at <math>\alpha = \pi/6</math>,
+
Dieser Ausdruck ist negativ für <math>\alpha = \pi/6</math>.
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}
A''(\pi/6) &= -100\cos\frac{\pi}{6}\cdot \Bigl(1+4\sin\frac{\pi}{6}\Bigr)\\[5pt]
A''(\pi/6) &= -100\cos\frac{\pi}{6}\cdot \Bigl(1+4\sin\frac{\pi}{6}\Bigr)\\[5pt]
-
&= -100\cdot\frac{\sqrt{3}}{2}\cdot \Bigl( 1+4\cdot \frac{1}{2} \Bigr)<0\,\textrm{.}
+
&= -100\cdot\frac{\sqrt{3}}{2}\cdot \Bigl( 1+4\cdot \frac{1}{2} \Bigr)<0\,\textrm{}
\end{align}</math>}}
\end{align}</math>}}
-
There are no local maximum points other than <math>\alpha = \pi/6\,</math>, which must therefore also be a global maximum.
+
Also hat die Funktion an der Stelle <math>\alpha = \pi/6\,</math> ein globales Maximum, da es das einzige lokale Maximum ist.

Aktuelle Version

Der Kanal kann am meisten Wasser enthalten, wenn seine Querschnittsfläche am größten ist.

Indem wir den Querschnitt des Kanals in ein Rechteck und zwei Dreiecke aufteilen, können wir mit ein wenig Trigonometrie die Querschnittsfläche des Kanals berechnen.

Die Fläche ist

\displaystyle \begin{align}

A(\alpha) &= 10\cdot 10\cos\alpha + 2\cdot\frac{1}{2}\cdot 10\cos\alpha \cdot 10\sin\alpha\\[5pt] &= 100\cos \alpha (1+\sin\alpha)\,\textrm{.} \end{align}

Begrenzen wir den Winkel so, dass er zwischen \displaystyle 0 und \displaystyle \pi/2 liegt, erhalten wir die folgende Optimierungsaufgabe:

Maximiere \displaystyle A(\alpha) = 100\cos\alpha (1+\sin\alpha) wenn \displaystyle 0\le \alpha \le {\pi }/{2}\,.

Die Funktion A ist überall differenzierbar und die Fläche ist minimal wenn \displaystyle \alpha=0 oder wenn \displaystyle \alpha=\pi/2\ , also nimmt die Funktion ihr Maximum in einem stationären Punkt an.

Die Ableitung ist

\displaystyle \begin{align}

A'(\alpha) &= 100\cdot (-\sin\alpha)\cdot (1+\sin\alpha) + 100\cdot\cos\alpha \cdot \cos\alpha\\[5pt] &= -100\sin\alpha - 100\sin^2\!\alpha + 100\cos^2\!\alpha\,\textrm{.} \end{align}

Für stationäre Punkte ist \displaystyle A'(\alpha)=0\,. Das ergibt die Gleichung

\displaystyle \sin\alpha + \sin^2\!\alpha - \cos^2\!\alpha = 0\,.

Nachdem wir den Faktor -100 heraus gezogen haben, ersetzen wir \displaystyle \cos^2\!\alpha mit \displaystyle 1-\sin^2\!\alpha und erhalten eine Gleichung mit nur \displaystyle \sin\alpha\,-Termen.

\displaystyle \begin{align}

\sin\alpha + \sin^2\!\alpha - (1-\sin^2\!\alpha) &= 0\\[5pt] 2\sin^2\!\alpha + \sin\alpha - 1 &= 0\,\textrm{} \end{align}

Das ist eine quadratische Gleichung für \displaystyle \sin\alpha. Durch quadratische Ergänzung erhalten wir

\displaystyle \begin{align}

2\bigl(\sin\alpha + \tfrac{1}{4}\bigr)^{2} - 2\bigl(\tfrac{1}{4}\bigr)^2 - 1 &= 0\\[5pt] \bigl(\sin\alpha + \tfrac{1}{4}\bigr)^2 &= \frac{9}{16} \end{align}.

Wir erhalten weiter \displaystyle \sin\alpha = -\tfrac{1}{4}\pm \tfrac{3}{4}, also \displaystyle \sin \alpha = -1 oder \displaystyle \sin \alpha = \tfrac{1}{2}\,.

Der Fall, dass \displaystyle \sin \alpha =-1, ist nie erfüllt für \displaystyle 0\le \alpha \le \pi/2 und \displaystyle \sin \alpha = \tfrac{1}{2} gibt \displaystyle \alpha = \pi/6. Also ist \displaystyle \alpha = \pi/6\, ein stationärer Punkt.


Wir wissen von der Zeichnung her, dass die Fläche lokale Minima an den Stellen \displaystyle \alpha = 0 und \displaystyle \alpha = \pi/2 hat und die kritische Stelle \displaystyle \alpha = \pi/6\,. Daher muss die Funktion an der kritischen Stelle ein Maximum haben, wir zeigen dies mit der zweiten Ableitung.

\displaystyle \begin{align}

A''(\alpha) &= -100\cos\alpha - 100\cdot 2\sin\alpha\cdot\cos\alpha + 100\cdot 2\cos\alpha \cdot (-\sin\alpha)\\[5pt] &= -100\cos\alpha (1+4\sin\alpha)\,. \end{align}

Dieser Ausdruck ist negativ für \displaystyle \alpha = \pi/6.

\displaystyle \begin{align}

A''(\pi/6) &= -100\cos\frac{\pi}{6}\cdot \Bigl(1+4\sin\frac{\pi}{6}\Bigr)\\[5pt] &= -100\cdot\frac{\sqrt{3}}{2}\cdot \Bigl( 1+4\cdot \frac{1}{2} \Bigr)<0\,\textrm{} \end{align}

Also hat die Funktion an der Stelle \displaystyle \alpha = \pi/6\, ein globales Maximum, da es das einzige lokale Maximum ist.

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_1.3:5“