Lösung 3.2:6e

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
Aktuelle Version (16:42, 22. Aug. 2009) (bearbeiten) (rückgängig)
 
(Der Versionsvergleich bezieht 4 dazwischen liegende Versionen mit ein.)
Zeile 1: Zeile 1:
-
If we manage to write the numerator and denominator in polar form, we can then use the fact that when division is carried out in polar form it is particularly simple. Division means, namely, that the numerator's magnitude is divided by the denominator's magnitude and that the numerator's argument is subtracted from denominator's argument or, in written form,
+
Wenn wir den Zähler und den Nenner auf Polarform bringen, können wir die Division einfach ausführen, indem wir die Betrage dividieren und die Argumente subtrahieren.
-
{{Displayed math||<math>\frac{r_1(\cos\alpha+i\sin\alpha)}{r_2(\cos\beta+i\sin\beta)} = \frac{r_1}{r_2}\bigl(\cos (\alpha-\beta) + i\sin (\alpha-\beta)\bigr)\,\textrm{.}</math>}}
+
{{Abgesetzte Formel||<math>\frac{r_1(\cos\alpha+i\sin\alpha)}{r_2(\cos\beta+i\sin\beta)} = \frac{r_1}{r_2}\bigl(\cos (\alpha-\beta) + i\sin (\alpha-\beta)\bigr)\,\textrm{.}</math>}}
-
Thus, we determine the numerator's and denominator's polar forms:
+
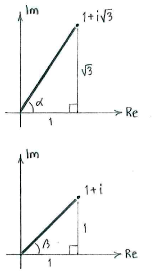
Daher schreiben wir den Zähler und den Nenner in Polarform:
[[Image:3_2_6_e_bild.gif]] [[Image:3_2_6_e_bildtext.gif]]
[[Image:3_2_6_e_bild.gif]] [[Image:3_2_6_e_bildtext.gif]]
-
Now, we obtain
+
und erhalten
-
{{Displayed math||<math>\begin{align}
+
{{Abgesetzte Formel||<math>\begin{align}
\frac{1+i\sqrt{3}}{1+i}
\frac{1+i\sqrt{3}}{1+i}
&= \frac{2\Bigl(\cos\dfrac{\pi}{3}+i\sin\dfrac{\pi}{3}\Bigr)}{\sqrt{2}\Bigl(\cos \dfrac{\pi}{4} + i\sin\dfrac{\pi}{4}\Bigr)}\\[5pt]
&= \frac{2\Bigl(\cos\dfrac{\pi}{3}+i\sin\dfrac{\pi}{3}\Bigr)}{\sqrt{2}\Bigl(\cos \dfrac{\pi}{4} + i\sin\dfrac{\pi}{4}\Bigr)}\\[5pt]

Aktuelle Version

Wenn wir den Zähler und den Nenner auf Polarform bringen, können wir die Division einfach ausführen, indem wir die Betrage dividieren und die Argumente subtrahieren.

\displaystyle \frac{r_1(\cos\alpha+i\sin\alpha)}{r_2(\cos\beta+i\sin\beta)} = \frac{r_1}{r_2}\bigl(\cos (\alpha-\beta) + i\sin (\alpha-\beta)\bigr)\,\textrm{.}

Daher schreiben wir den Zähler und den Nenner in Polarform:

Image:3_2_6_e_bild.gif Image:3_2_6_e_bildtext.gif

und erhalten

\displaystyle \begin{align}

\frac{1+i\sqrt{3}}{1+i} &= \frac{2\Bigl(\cos\dfrac{\pi}{3}+i\sin\dfrac{\pi}{3}\Bigr)}{\sqrt{2}\Bigl(\cos \dfrac{\pi}{4} + i\sin\dfrac{\pi}{4}\Bigr)}\\[5pt] &= \frac{2}{\sqrt{2}}\Bigl( \cos\Bigl(\frac{\pi}{3}-\frac{\pi}{4}\Bigr) + i\sin\Bigl( \frac{\pi}{3}-\frac{\pi}{4}\Bigr)\Bigr)\\[5pt] &= \sqrt{2}\Bigl(\cos\frac{\pi}{12}+i\sin\frac{\pi}{12}\Bigr)\,\textrm{.} \end{align}

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.2:6e“