Lösung 3.2:5a

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
Aktuelle Version (17:25, 14. Sep. 2009) (bearbeiten) (rückgängig)
 
(Der Versionsvergleich bezieht 3 dazwischen liegende Versionen mit ein.)
Zeile 1: Zeile 1:
-
The argument of a complex number is angle of the line between the origin and the number measured with respect the positive real number axis.
+
Das Argument einer komplexen Zahl ist der Winkel zwischen der Zahl und der positiven reellen Achse, gegen den Uhrzeigersinn.
-
 
+
-
In this case, we see directly that
+
-
<math>-\text{1}0</math>
+
-
has the argument
+
-
<math>\pi </math>.
+
 +
In diesem Fall sehen wir direkt dass das Winkel von <math>-10</math>, <math>\pi</math> ist.
[[Image:3_2_5_a.gif|center]]
[[Image:3_2_5_a.gif|center]]
-
 
+
Hinweis: Alle Argumente die sich nur mit ein Vielfaches von <math>2\pi</math> unterscheiden, beschreiben bekanntlich denselben Winkel. Also hätten wir auch mit <math>-\pi</math>, <math>3\pi</math>, <math>5\pi</math> etc antworten können. Meistens antwortet man aber mit einen Winkel entweder zwischen <math>0</math> und <math>2\pi</math> oder zwischen <math>-\pi</math> und <math>\pi</math>.
-
NOTE: All angles that correspond to the same direction differ by a whole number of turns, i.e. a multiple of
+
-
<math>2\pi </math>, and therefore we could have just as well answered
+
-
<math>-\pi </math>,
+
-
<math>3\pi </math>,
+
-
<math>5\pi </math>
+
-
etc. However, it is usual to give the argument between
+
-
<math>0</math>
+
-
and
+
-
<math>2\pi </math>
+
-
or between
+
-
<math>-\pi </math>
+
-
and
+
-
<math>\pi </math>.
+

Aktuelle Version

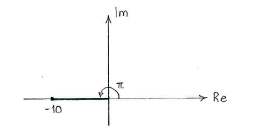
Das Argument einer komplexen Zahl ist der Winkel zwischen der Zahl und der positiven reellen Achse, gegen den Uhrzeigersinn.

In diesem Fall sehen wir direkt dass das Winkel von \displaystyle -10, \displaystyle \pi ist.

Hinweis: Alle Argumente die sich nur mit ein Vielfaches von \displaystyle 2\pi unterscheiden, beschreiben bekanntlich denselben Winkel. Also hätten wir auch mit \displaystyle -\pi, \displaystyle 3\pi, \displaystyle 5\pi etc antworten können. Meistens antwortet man aber mit einen Winkel entweder zwischen \displaystyle 0 und \displaystyle 2\pi oder zwischen \displaystyle -\pi und \displaystyle \pi.

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.2:5a“