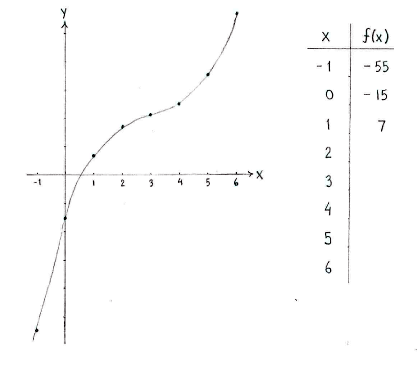Lösung 1.3:2d

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
Aktuelle Version (11:27, 9. Sep. 2009) (bearbeiten) (rückgängig)
 
(Der Versionsvergleich bezieht 8 dazwischen liegende Versionen mit ein.)
Zeile 1: Zeile 1:
-
If the function has several local extreme points, then they must lie between the following three types of points:
+
Lokale Extremstellen einer Funktion sind entweder:
-
1. Critical points, i.e. where
+
# stationäre Stellen mit <math>f^{\,\prime}(x)=0</math>,
-
<math>{f}'\left( x \right)=0</math>;
+
# singuläre Stellen, in denen die Funktion nicht differenzierbarbar ist, oder
 +
# Randstellen.
 +
 +
Da die Funktion ein Polynom ist, ist sie überall definiert und überall differenzierbar. Es gibt also keine Extremstellen, die die Bedienungen 2 und 3 erfüllen.
-
2. Points where the function is not differentiable;
+
Die Ableitung ist
-
3. Endpoints of the interval of definition.
+
{{Abgesetzte Formel||<math>f^{\,\prime}(x) = 3x^2 - 18x + 30 = 3(x^2-6x+10)</math>}}
-
2. and 3. do not give any points, because our function, which is a polynomial, is defined and differentiable everywhere. In order to investigate if there are any critical points, we set the derivative,
+
und wir erhalten die Gleichung
 +
{{Abgesetzte Formel||<math>x^{2}-6x+10=0\,\textrm{.}</math>}}
-
<math>{f}'\left( x \right)=3x^{2}-18x+30=3\left( x^{2}-6x+10 \right)</math>
+
Die quadratische Ergänzung ergibt
 +
{{Abgesetzte Formel||<math>(x-3)^2 - 3^2 + 10 = 0\,,</math>}}
-
equal to zero and obtain the equation
+
also
 +
{{Abgesetzte Formel||<math>(x-3)^2 + 1 = 0\,\textrm{.}</math>}}
-
<math>x^{2}-6x+10=0</math>
+
Diese Gleichung hat keine Lösung, also hat die Funktion keine lokalen Extremstellen. Bei der Ableitung
 +
{{Abgesetzte Formel||<math>f^{\,\prime}(x) = 3((x-3)^2+1)</math>}}
-
Completing the square gives
+
sehen wir, dass sie immer größer als null ist, also ist die Funktion streng monoton steigend. Wir berechnen einige Funktionswerte, um die Funktion zu zeichnen.
-
 
+
-
 
+
-
<math>\left( x-3 \right)^{2}-3^{2}+10=0</math>
+
-
 
+
-
 
+
-
i.e.
+
-
 
+
-
 
+
-
<math>\left( x-3 \right)^{2}+1=0</math>
+
-
 
+
-
 
+
-
This equation does not have any real roots, because the left-hand side is always greater than or equal to
+
-
<math>\text{1}</math>, regardless of how
+
-
<math>x</math>
+
-
is chosen (the square
+
-
<math>\left( x-3 \right)^{2}</math>
+
-
can never be negative).
+
-
 
+
-
This means that the function does not have any local extreme points.
+
-
 
+
-
From the derivative's appearance,
+
-
 
+
-
 
+
-
<math>{f}'\left( x \right)=3\left( \left( x-3 \right)^{2}+1 \right)</math>
+
-
 
+
-
 
+
-
we see that it is always greater than zero and therefore that the function is strictly increasing. We do not have so much more information when we sketch the graph of the function, other than the function's value at a few points.
+
-
 
+
-
PICTURE GRAPH
+
-
 
+
[[Image:1_3_2_d.gif|center]]
[[Image:1_3_2_d.gif|center]]

Aktuelle Version

Lokale Extremstellen einer Funktion sind entweder:

  1. stationäre Stellen mit \displaystyle f^{\,\prime}(x)=0,
  2. singuläre Stellen, in denen die Funktion nicht differenzierbarbar ist, oder
  3. Randstellen.

Da die Funktion ein Polynom ist, ist sie überall definiert und überall differenzierbar. Es gibt also keine Extremstellen, die die Bedienungen 2 und 3 erfüllen.

Die Ableitung ist

\displaystyle f^{\,\prime}(x) = 3x^2 - 18x + 30 = 3(x^2-6x+10)

und wir erhalten die Gleichung

\displaystyle x^{2}-6x+10=0\,\textrm{.}

Die quadratische Ergänzung ergibt

\displaystyle (x-3)^2 - 3^2 + 10 = 0\,,

also

\displaystyle (x-3)^2 + 1 = 0\,\textrm{.}

Diese Gleichung hat keine Lösung, also hat die Funktion keine lokalen Extremstellen. Bei der Ableitung

\displaystyle f^{\,\prime}(x) = 3((x-3)^2+1)

sehen wir, dass sie immer größer als null ist, also ist die Funktion streng monoton steigend. Wir berechnen einige Funktionswerte, um die Funktion zu zeichnen.

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_1.3:2d“