Lösung 3.2:4a
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
| (Der Versionsvergleich bezieht 4 dazwischen liegende Versionen mit ein.) | |||
| Zeile 1: | Zeile 1: | ||
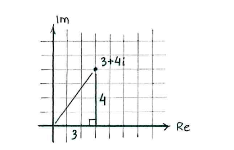
| - | + | Der Betrag von <math>3+4i</math> ist der Abstand zum Punkt <math>(0,0)</math> in der komplexen Zahlenebene. | |
| - | <math> | + | |
| - | + | ||
| - | + | Durch den Satz des Pythagoras erhalten wir den Abstand | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| + | {{Abgesetzte Formel||<math>|3+4i| = \sqrt{3^2+4^2} = \sqrt{9+16} = \sqrt{25} = 5\,\textrm{.}</math>}} | ||
[[Image:3_2_4_a.gif|center]] | [[Image:3_2_4_a.gif|center]] | ||
| - | NOTE: In general, the magnitude of a complex number | ||
| - | <math>z=x+iy</math> | ||
| - | is equal to | ||
| + | Hinweis: Allgemein ist der Betrag einer komplexen Zahl <math>z=x+iy</math>, | ||
| - | <math> | + | {{Abgesetzte Formel||<math>|z| = |x+iy| = \sqrt{x^2+y^2}\,\textrm{.}</math>}} |
Aktuelle Version
Der Betrag von \displaystyle 3+4i ist der Abstand zum Punkt \displaystyle (0,0) in der komplexen Zahlenebene.
Durch den Satz des Pythagoras erhalten wir den Abstand
| \displaystyle |3+4i| = \sqrt{3^2+4^2} = \sqrt{9+16} = \sqrt{25} = 5\,\textrm{.} |
Hinweis: Allgemein ist der Betrag einer komplexen Zahl \displaystyle z=x+iy,
| \displaystyle |z| = |x+iy| = \sqrt{x^2+y^2}\,\textrm{.} |