Aus Online Mathematik Brückenkurs 2

Version vom 09:23, 29. Okt. 2008 (bearbeiten) Tek (Diskussion | Beiträge) K ← Zum vorherigen Versionsunterschied		Aktuelle Version (15:54, 22. Aug. 2009) (bearbeiten) (rückgängig) Moni (Diskussion | Beiträge)
(Der Versionsvergleich bezieht 3 dazwischen liegende Versionen mit ein.)
Zeile 1:		Zeile 1:
-	By calculation, we obtain	+	Durch Rechnung erhalten wir
-	{{Displayed math||<math>2z+w = 2(2+i)+(2+3i) = 2\cdot 2 + 2 + (2+3)i = 6+5i</math>}}	+	{{Abgesetzte Formel||<math>2z+w = 2(2+i)+(2+3i) = 2\cdot 2 + 2 + (2+3)i = 6+5i</math>}}
-	and we can mark this point on the complex plane.	+	Diesen Punkt können wir in der komplexen Zahlenebene einzeichnen.
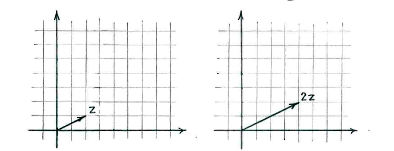
-	If we treat <math>z</math> and <math>w</math> as vectors, then <math>2z</math> is the vector which has the same direction as <math>z</math>, but is twice as long.	+	Wenn wir <math>z</math> und <math>w</math> als Vektoren sehen, ist <math>2z</math> doppelt so lang, aber hat dieselbe Richtung wie <math>z</math>.
	[[Image:3_2_1_c1.gif|center]]		[[Image:3_2_1_c1.gif|center]]
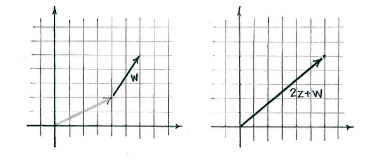
-	We add <math>w</math> to this vector and get <math>2z+w</math>.	+	Wir addieren <math>w</math> zu diesen Vektor und erhalten <math>2z+w</math>.
	[[Image:3_2_1_c2.gif|center]]		[[Image:3_2_1_c2.gif|center]]

---

Aktuelle Version

Durch Rechnung erhalten wir

\displaystyle 2z+w = 2(2+i)+(2+3i) = 2\cdot 2 + 2 + (2+3)i = 6+5i

Diesen Punkt können wir in der komplexen Zahlenebene einzeichnen.

Wenn wir \displaystyle z und \displaystyle w als Vektoren sehen, ist \displaystyle 2z doppelt so lang, aber hat dieselbe Richtung wie \displaystyle z.

Wir addieren \displaystyle w zu diesen Vektor und erhalten \displaystyle 2z+w.