Lösung 3.2:5b
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
K (Solution 3.2:5b moved to Lösung 3.2:5b: Robot: moved page) |
Version vom 10:39, 11. Mär. 2009
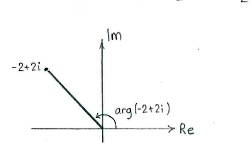
The number \displaystyle -2+2i lies in the second quadrant and if we use an auxiliary triangle in that quadrant (according to the figure), we can use simple trigonometry to determine the angle \displaystyle \alpha which the line between the origin and \displaystyle -2+2i makes with the positive imaginary axis.
The argument of \displaystyle -2+2i, which is the angle to the positive real axis, therefore becomes
| \displaystyle \arg (-2+2i) = \frac{\pi}{2} + \alpha = \frac{\pi}{2} + \frac{\pi}{4} = \frac{3\pi}{4}\,\textrm{.} |