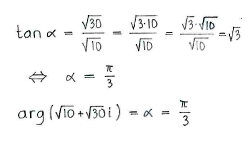Lösung 3.2:6d
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K |
|||
| Zeile 1: | Zeile 1: | ||
| - | We can determine the number's magnitude directly using the distance formula | + | We can determine the number's magnitude directly using the distance formula, |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| + | {{Displayed math||<math>\begin{align} | ||
| + | \bigl|\sqrt{10}+\sqrt{30}i\bigr| | ||
| + | &= \sqrt{\bigl(\sqrt{10}\,\bigr)^2+\bigl(\sqrt{30}\,\bigr)^2}\\[5pt] | ||
| + | &= \sqrt{10+30}\\[5pt] | ||
| + | &= \sqrt{40}\\[5pt] | ||
| + | &= \sqrt{4\cdot 10}\\[5pt] | ||
| + | &= 2\sqrt{10}\,\textrm{.} | ||
| + | \end{align}</math>}} | ||
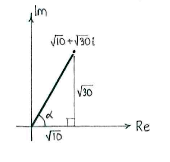
In addition, the number lies in the first quadrant and we can therefore determine the argument using simple trigonometry. | In addition, the number lies in the first quadrant and we can therefore determine the argument using simple trigonometry. | ||
| - | |||
[[Image:3_2_6_d_bild.gif]] [[Image:3_2_6_d_bildtext.gif]] | [[Image:3_2_6_d_bild.gif]] [[Image:3_2_6_d_bildtext.gif]] | ||
| - | |||
The polar form is | The polar form is | ||
| - | + | {{Displayed math||<math>2\sqrt{10}\Bigl( \cos\frac{\pi}{3} + i\sin\frac{\pi}{3} \Bigr)\,\textrm{.}</math>}} | |
| - | <math>2\sqrt{10}\ | + | |
Version vom 13:56, 29. Okt. 2008
We can determine the number's magnitude directly using the distance formula,
| \displaystyle \begin{align}
\bigl|\sqrt{10}+\sqrt{30}i\bigr| &= \sqrt{\bigl(\sqrt{10}\,\bigr)^2+\bigl(\sqrt{30}\,\bigr)^2}\\[5pt] &= \sqrt{10+30}\\[5pt] &= \sqrt{40}\\[5pt] &= \sqrt{4\cdot 10}\\[5pt] &= 2\sqrt{10}\,\textrm{.} \end{align} |
In addition, the number lies in the first quadrant and we can therefore determine the argument using simple trigonometry.
The polar form is
| \displaystyle 2\sqrt{10}\Bigl( \cos\frac{\pi}{3} + i\sin\frac{\pi}{3} \Bigr)\,\textrm{.} |