Lösung 3.2:3
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K |
|||
| Zeile 1: | Zeile 1: | ||
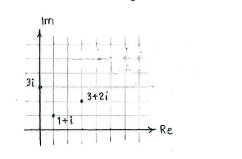
| - | If we mark the three complex numbers in the plane, we see that the fourth corner will have | + | If we mark the three complex numbers in the plane, we see that the fourth corner will have <math>3+2i</math> and <math>3i</math> as neighbouring corners. |
| - | <math> | + | |
| - | and | + | |
| - | <math> | + | |
| - | as neighbouring corners. | + | |
| - | + | ||
[[Image:3_2_3_1.gif|center]] | [[Image:3_2_3_1.gif|center]] | ||
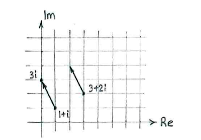
| - | In order to find the fourth corner, we use the fact that in a square opposite sides are parallel and all sides have the same length. This means that the vector from | + | In order to find the fourth corner, we use the fact that in a square opposite sides are parallel and all sides have the same length. This means that the vector from <math>1+i</math> to <math>3i</math> is equal to the vector from <math>3+2i</math> to the fourth corner. |
| - | <math> | + | |
| - | to | + | |
| - | <math> | + | |
| - | is equal to the vector from | + | |
| - | <math> | + | |
| - | to the fourth corner. | + | |
| - | + | ||
[[Image:3_2_3_2.gif|center]] | [[Image:3_2_3_2.gif|center]] | ||
If we interpret the complex numbers as vectors, this means that the vector from | If we interpret the complex numbers as vectors, this means that the vector from | ||
| - | <math> | + | <math>1+i</math> to <math>\text{3}i</math> is |
| - | to | + | |
| - | <math>\text{3}i</math> | + | |
| - | is | + | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | {{Displayed math||<math>3i-(1+i) = -1+2i</math>}} | |
| - | <math> | + | |
| + | and we obtain the fourth corner if we add this vector to the corner <math>3+2i</math>, | ||
| - | <math> | + | {{Displayed math||<math>3+2i+(-1+2i) = 2+4i\,\textrm{.}</math>}} |
Version vom 09:51, 29. Okt. 2008
If we mark the three complex numbers in the plane, we see that the fourth corner will have \displaystyle 3+2i and \displaystyle 3i as neighbouring corners.
In order to find the fourth corner, we use the fact that in a square opposite sides are parallel and all sides have the same length. This means that the vector from \displaystyle 1+i to \displaystyle 3i is equal to the vector from \displaystyle 3+2i to the fourth corner.
If we interpret the complex numbers as vectors, this means that the vector from \displaystyle 1+i to \displaystyle \text{3}i is
| \displaystyle 3i-(1+i) = -1+2i |
and we obtain the fourth corner if we add this vector to the corner \displaystyle 3+2i,
| \displaystyle 3+2i+(-1+2i) = 2+4i\,\textrm{.} |