Lösung 2.1:4d

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
K
Zeile 3: Zeile 3:
[[Image:2_1_4_d1.gif|center]]
[[Image:2_1_4_d1.gif|center]]
-
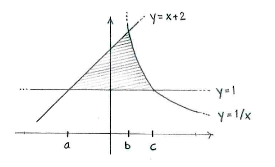
When we draw the curves on the same diagram, we see that the region is bounded from below in the
+
When we draw the curves on the same diagram, we see that the region is bounded from below in the ''y''-direction by the horizontal line <math>y=1</math> and above partly by <math>y=x+2</math> and partly by <math>y=1/x\,</math>.
-
<math>y</math>
+
-
-direction by the horizontal line
+
-
<math>y=\text{1}</math>
+
-
and above partly by
+
-
<math>y=x+2</math>
+
-
and partly by
+
-
<math>y=\frac{1}{x}</math>.
+
-
 
+
[[Image:2_1_4_d2.gif|center]]
[[Image:2_1_4_d2.gif|center]]
-
If we denote the
+
If we denote the ''x''-coordinates of the intersection points between the curves by <math>x=a</math>, <math>x=b</math> and <math>x=c</math>, as shown in the figure, we see that the region can be divided up into two parts. In the left part between <math>x=a</math> and <math>x=b</math>, the upper limit is <math>y=x+2</math>, whilst in the right part between <math>x=b</math> and <math>x=c</math> the curve <math>y=1/x</math> is the upper limit.
-
<math>x</math>
+
-
-coordinates of the intersection points by
+
-
<math>x=a</math>,
+
-
<math>x=b</math>
+
-
and
+
-
<math>x=c</math>, as shown in the figure, we see that the region can be divided up into two parts. In the left part between
+
-
<math>x=a</math>
+
-
and
+
-
<math>x=b</math>, the upper limit is
+
-
<math>y=x+2</math>, whilst in the right part between
+
-
<math>x=b</math>
+
-
and
+
-
<math>x=c</math>
+
-
+
-
<math>y={1}/{x}\;</math>
+
-
is the upper limit.
+
-
 
+
[[Image:2_1_4_d3.gif|center]]
[[Image:2_1_4_d3.gif|center]]
Zeile 38: Zeile 13:
The area of each part is given by the integrals
The area of each part is given by the integrals
-
Left area
+
{{Displayed math||<math>\begin{align}
-
<math>=\int\limits_{a}^{b}{\left( x+2-1 \right)}\,dx</math>
+
\text{Left area} &= \int\limits_a^b (x+2-1)\,dx\,,\\[5pt]
-
 
+
\text{Right area} &= \int\limits_b^c \Bigl(\frac{1}{x}-1\Bigr)\,dx\,,
-
 
+
\end{align}</math>}}
-
Right area
+
-
<math>=\int\limits_{b}^{c}{\left( \frac{1}{x}-1 \right)}\,dx</math>
+
-
 
+
and the total area is the sum of these areas.
and the total area is the sum of these areas.
Zeile 52: Zeile 24:
To determine the points of intersection:
To determine the points of intersection:
 +
*<math>x=a</math>: The point of intersection between <math>y=1</math> and <math>y=x+2</math> must satisfy both equations of the lines,
-
<math>x=a</math>: the point of intersection between
+
{{Displayed math||<math>\left\{\begin{align}
-
<math>y=\text{1 }</math>
+
y &= 1\,,\\[5pt]
-
and
+
y &= x+2\,\textrm{.}
-
<math>y=x+\text{2}</math>
+
\end{align}\right.</math>}}
-
must both satisfy the equations of the lines
+
 +
:This gives that <math>x</math> must satisfy <math>x+2=1</math>, i.e. <math>x=-1\,</math>. Thus, <math>a=-1\,</math>.
-
<math>\left\{ \begin{array}{*{35}l}
 
-
y=\text{1} \\
 
-
y=x+\text{2} \\
 
-
\end{array} \right.</math>
 
 +
*<math>x=b</math>: At the point where the curves <math>y=x+2</math> and <math>y=1/x</math> meet, we have that
-
This gives that
+
{{Displayed math||<math>\left\{\begin{align}
-
<math>x</math>
+
y &= x+2\,,\\[5pt]
-
must satisfy
+
y &= 1/x\,\textrm{.}
-
<math>x+\text{2}=\text{1}</math>, i.e.
+
\end{align}\right.</math>}}
-
<math>x=-\text{1 }</math>
+
-
Thus,
+
-
<math>a=-\text{1}</math>.
+
 +
:If we eliminate <math>y</math>, we obtain an equation for <math>x</math>,
-
<math>x=b</math>: At the point where the curves
+
{{Displayed math||<math>x+2=\frac{1}{x}\,,</math>}}
-
<math>y=x+\text{2}</math>
+
-
and
+
-
<math>y={1}/{x}\;</math>
+
-
meet, we have that
+
 +
:which we multiply by <math>x</math>,
-
<math>\left\{ \begin{array}{*{35}l}
+
{{Displayed math||<math>x^{2}+2x=1\,\textrm{.}</math>}}
-
y=x+\text{2} \\
+
-
y={1}/{x}\; \\
+
-
\end{array} \right.</math>
+
 +
:Completing the square of the left-hand side,
-
If we eliminate
+
{{Displayed math||<math>\begin{align}
-
<math>y</math>, we obtain an equation for
+
(x+1)^2 - 1^2 &= 1\,,\\[5pt]
-
<math>x</math>,
+
(x+1)^2 &= 2\,,
 +
\end{align}</math>}}
 +
:and taking the square root gives that <math>x=-1\pm \sqrt{2}</math>, leading to
 +
<math>b=-1+\sqrt{2}</math>. (The alternative <math>b=-1-\sqrt{2}</math>
 +
lies to the left of <math>x=a\,</math>.)
-
<math>x+2=\frac{1}{x}</math>,
 
-
which we multiply by
+
*<math>x=c</math>: The final point of intersection is given by the condition that the equation to both curves, <math>y=1</math> and <math>y=1/x\,</math>, are satisfied simultaneously. We see almost immediately that this gives <math>x=1\,</math>, i.e. <math>c=1\,</math>.
-
<math>x</math>,
+
- 
-
<math>x^{2}+2x=1</math>
 
- 
- 
-
Completing the square of the left-hand side,
 
- 
- 
-
<math>\begin{align}
 
-
& \left( x+1 \right)^{2}-1^{2}=1 \\
 
-
& \left( x+1 \right)^{2}=2 \\
 
-
\end{align}</math>,
 
- 
-
and taking the root gives that
 
-
<math>x=-1\pm \sqrt{2}</math>, leading to
 
-
<math>b=-1+\sqrt{2}</math>
 
-
(the alternative
 
-
<math>b=-1-\sqrt{2}</math>
 
-
lies to the left of
 
-
<math>b=a</math>).
 
- 
- 
-
<math>x=c</math>: the final point of intersection is given by the condition that the equation to both curves,
 
-
<math>y=\text{1}</math>
 
-
and
 
-
<math>y={1}/{x}\;</math>, are satisfied simultaneously. We see almost immediately that this gives
 
-
<math>x=\text{1}</math>, i.e.
 
-
<math>c=\text{ 1}</math>.
 
The sub-areas are
The sub-areas are
-
 
+
{{Displayed math||<math>\begin{align}
-
<math>\begin{align}
+
\text{Left area}
-
& \text{Leftarea }=\text{ }\int\limits_{-1}^{\sqrt{2}-1}{\left( x+2-1 \right)}\,dx=\int\limits_{-1}^{\sqrt{2}-1}{\left( x+1 \right)}\,dx \\
+
&= \int\limits_{-1}^{\sqrt{2}-1} (x+2-1)\,dx\\[5pt]
-
& =\left[ \frac{x^{2}}{2}+x \right]_{-1}^{\sqrt{2}-1} \\
+
&= \int\limits_{-1}^{\sqrt{2}-1} (x+1)\,dx\\[5pt]
-
& =\frac{\left( \sqrt{2}-1 \right)^{2}}{2}+\sqrt{2}-1-\left( \frac{\left( -1 \right)^{2}}{2}+\left( -1 \right) \right) \\
+
&= \Bigl[\ \frac{x^2}{2} + x\ \Bigr]_{-1}^{\sqrt{2}-1}\\[5pt]
-
& =\frac{\left( \sqrt{2} \right)^{2}-2\sqrt{2}+1}{2}+\sqrt{2}-1-\frac{1}{2}+1 \\
+
&= \frac{\bigl(\sqrt{2}-1\bigr)^2}{2} + \sqrt{2} - 1 - \Bigl(\frac{(-1)^2}{2} + (-1) \Bigr)\\[5pt]
-
& =\frac{2-2\sqrt{2}+1}{2}+\sqrt{2}-1-\frac{1}{2}+1 \\
+
&= \frac{\bigl(\sqrt{2}\bigr)^2-2\sqrt{2}+1}{2} + \sqrt{2} - 1 - \frac{1}{2} + 1\\[5pt]
-
& =1-\sqrt{2}+\frac{1}{2}+\sqrt{2}-1-\frac{1}{2}+1 \\
+
&= \frac{2-2\sqrt{2}+1}{2} + \sqrt{2} - 1 - \frac{1}{2} + 1\\[5pt]
-
& =1 \\
+
&= 1 - \sqrt{2} + \frac{1}{2} + \sqrt{2} - 1 - \frac{1}{2} + 1\\[5pt]
-
\end{align}</math>
+
&= 1\,,\\[10pt]
-
 
+
\text{Right area}
-
 
+
&= \int\limits_{\sqrt{2}-1}^1 \Bigl(\frac{1}{x}-1\Bigr)\,dx\\[5pt]
-
 
+
&= \Bigl[\ \ln |x| - x\ \Bigr]_{\sqrt{2}-1}^1\\[5pt]
-
<math>\begin{align}
+
&= \ln 1 - 1 - \Bigl( \ln \bigl(\sqrt{2}-1\bigr)-\bigl(\sqrt{2}-1\bigr)\Bigr)\\[5pt]
-
& \text{Rightarea }=\int\limits_{\sqrt{2}-1}^{1}{\left( \frac{1}{x}-1 \right)}\,dx=\left[ \ln \left| x \right|-x \right]_{\sqrt{2}-1}^{1} \\
+
&= 0 - 1 - \ln \bigl(\sqrt{2}-1\bigr) + \sqrt{2} - 1\\[5pt]
-
& =\ln 1-1-\left( \ln \left( \sqrt{2}-1 \right)-\left( \sqrt{2}-1 \right) \right) \\
+
&= \sqrt{2} - 2 - \ln\bigl(\sqrt{2}-1\bigr)\,\textrm{.}
-
& =0-1-\ln \left( \sqrt{2}-1 \right)+\sqrt{2}-1 \\
+
\end{align}</math>}}
-
& =\sqrt{2}-2-\ln \left( \sqrt{2}-1 \right) \\
+
-
\end{align}</math>
+
-
 
+
The total area is
The total area is
-
Area = (left area +right area)
+
{{Displayed math||<math>\begin{align}
-
+
\text{Area}
-
<math>\begin{align}
+
&= \text{(left area)} + \text{(right area)}\\[5pt]
-
& =1+\sqrt{2}-2-\ln \left( \sqrt{2}-1 \right) \\
+
&= 1 + \sqrt{2} - 2 - \ln\bigl(\sqrt{2}-1\bigr)\\[5pt]
-
& =\sqrt{2}-1-\ln \left( \sqrt{2}-1 \right) \\
+
&= \sqrt{2} - 1 - \ln\bigl(\sqrt{2}-1\bigr)\,\textrm{.}
-
\end{align}</math>
+
\end{align}</math>}}

Version vom 09:04, 28. Okt. 2008

We start by drawing the three curves:

When we draw the curves on the same diagram, we see that the region is bounded from below in the y-direction by the horizontal line \displaystyle y=1 and above partly by \displaystyle y=x+2 and partly by \displaystyle y=1/x\,.

If we denote the x-coordinates of the intersection points between the curves by \displaystyle x=a, \displaystyle x=b and \displaystyle x=c, as shown in the figure, we see that the region can be divided up into two parts. In the left part between \displaystyle x=a and \displaystyle x=b, the upper limit is \displaystyle y=x+2, whilst in the right part between \displaystyle x=b and \displaystyle x=c the curve \displaystyle y=1/x is the upper limit.

The area of each part is given by the integrals

\displaystyle \begin{align}

\text{Left area} &= \int\limits_a^b (x+2-1)\,dx\,,\\[5pt] \text{Right area} &= \int\limits_b^c \Bigl(\frac{1}{x}-1\Bigr)\,dx\,, \end{align}

and the total area is the sum of these areas.

If we just manage to determine the curves' points of intersection, the rest is just a matter of integration.

To determine the points of intersection:

  • \displaystyle x=a: The point of intersection between \displaystyle y=1 and \displaystyle y=x+2 must satisfy both equations of the lines,
\displaystyle \left\{\begin{align}

y &= 1\,,\\[5pt] y &= x+2\,\textrm{.} \end{align}\right.

This gives that \displaystyle x must satisfy \displaystyle x+2=1, i.e. \displaystyle x=-1\,. Thus, \displaystyle a=-1\,.


  • \displaystyle x=b: At the point where the curves \displaystyle y=x+2 and \displaystyle y=1/x meet, we have that
\displaystyle \left\{\begin{align}

y &= x+2\,,\\[5pt] y &= 1/x\,\textrm{.} \end{align}\right.

If we eliminate \displaystyle y, we obtain an equation for \displaystyle x,
\displaystyle x+2=\frac{1}{x}\,,
which we multiply by \displaystyle x,
\displaystyle x^{2}+2x=1\,\textrm{.}
Completing the square of the left-hand side,
\displaystyle \begin{align}

(x+1)^2 - 1^2 &= 1\,,\\[5pt] (x+1)^2 &= 2\,, \end{align}

and taking the square root gives that \displaystyle x=-1\pm \sqrt{2}, leading to

\displaystyle b=-1+\sqrt{2}. (The alternative \displaystyle b=-1-\sqrt{2} lies to the left of \displaystyle x=a\,.)


  • \displaystyle x=c: The final point of intersection is given by the condition that the equation to both curves, \displaystyle y=1 and \displaystyle y=1/x\,, are satisfied simultaneously. We see almost immediately that this gives \displaystyle x=1\,, i.e. \displaystyle c=1\,.


The sub-areas are

\displaystyle \begin{align}

\text{Left area} &= \int\limits_{-1}^{\sqrt{2}-1} (x+2-1)\,dx\\[5pt] &= \int\limits_{-1}^{\sqrt{2}-1} (x+1)\,dx\\[5pt] &= \Bigl[\ \frac{x^2}{2} + x\ \Bigr]_{-1}^{\sqrt{2}-1}\\[5pt] &= \frac{\bigl(\sqrt{2}-1\bigr)^2}{2} + \sqrt{2} - 1 - \Bigl(\frac{(-1)^2}{2} + (-1) \Bigr)\\[5pt] &= \frac{\bigl(\sqrt{2}\bigr)^2-2\sqrt{2}+1}{2} + \sqrt{2} - 1 - \frac{1}{2} + 1\\[5pt] &= \frac{2-2\sqrt{2}+1}{2} + \sqrt{2} - 1 - \frac{1}{2} + 1\\[5pt] &= 1 - \sqrt{2} + \frac{1}{2} + \sqrt{2} - 1 - \frac{1}{2} + 1\\[5pt] &= 1\,,\\[10pt] \text{Right area} &= \int\limits_{\sqrt{2}-1}^1 \Bigl(\frac{1}{x}-1\Bigr)\,dx\\[5pt] &= \Bigl[\ \ln |x| - x\ \Bigr]_{\sqrt{2}-1}^1\\[5pt] &= \ln 1 - 1 - \Bigl( \ln \bigl(\sqrt{2}-1\bigr)-\bigl(\sqrt{2}-1\bigr)\Bigr)\\[5pt] &= 0 - 1 - \ln \bigl(\sqrt{2}-1\bigr) + \sqrt{2} - 1\\[5pt] &= \sqrt{2} - 2 - \ln\bigl(\sqrt{2}-1\bigr)\,\textrm{.} \end{align}

The total area is

\displaystyle \begin{align}

\text{Area} &= \text{(left area)} + \text{(right area)}\\[5pt] &= 1 + \sqrt{2} - 2 - \ln\bigl(\sqrt{2}-1\bigr)\\[5pt] &= \sqrt{2} - 1 - \ln\bigl(\sqrt{2}-1\bigr)\,\textrm{.} \end{align}

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_2.1:4d“