Lösung 2.1:1d
Aus Online Mathematik Brückenkurs 2
K |
|||
| Zeile 1: | Zeile 1: | ||
| - | The modulus function, | + | The modulus function, <math>|x|</math>, strips <math>x</math> of its sign, e.g. |
| - | <math> | + | |
| - | <math>x</math> | + | |
| - | of its sign, e.g. | + | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| + | {{Displayed math||<math>|-5|=5\,</math>, <math>\quad|3|=3\quad</math> and <math>\quad |-\pi|=\pi\,</math>.}} | ||
| + | For positive values of <math>x</math>, the modulus function has no effect, since | ||
| + | <math>|x|=x</math>, but for negative <math>x</math> the modulus function changes the sign of <math>x</math>, i.e. <math>|x|=-x</math> (remember that <math>x</math> | ||
| + | is negative and therefore <math>-x</math> is positive). | ||
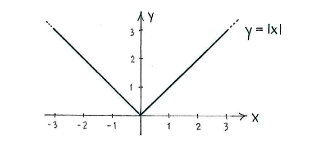
| + | If we draw a graph of <math>y=|x|</math> it will consist of two parts. For | ||
| + | <math>x\ge 0</math> we have <math>y=x</math>, and for <math>x\le 0</math> we have | ||
| + | <math>y=-x\,</math>. | ||
[[Image:2_1_1_d1.gif|center]] | [[Image:2_1_1_d1.gif|center]] | ||
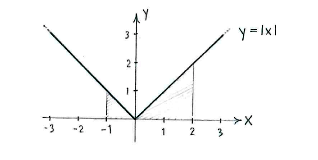
| - | + | The value of the integral is the area of the region under the graph <math>y=|x|</math> and between <math>x=-1</math> and <math>x=2</math>. | |
| - | The value of the integral is the area of the region under the graph | + | |
| - | <math>y= | + | |
| - | and between | + | |
| - | <math>x=-1</math> | + | |
| - | and | + | |
| - | <math>x=2</math>. | + | |
| - | + | ||
[[Image:2_1_1_d2.gif|center]] | [[Image:2_1_1_d2.gif|center]] | ||
| - | |||
This region consists of two triangles and we therefore obtain | This region consists of two triangles and we therefore obtain | ||
| - | <math>\int\limits_{-1}^{2} | + | {{Displayed math||<math>\int\limits_{-1}^{2} |x|\,dx = \frac{1}{2}\cdot 1\cdot 1 + \frac{1}{2}\cdot 2\cdot 2 = \frac{5}{2}\,\textrm{.}</math>}} |
Version vom 12:25, 21. Okt. 2008
The modulus function, \displaystyle |x|, strips \displaystyle x of its sign, e.g.
| \displaystyle |-5|=5\,, \displaystyle \quad|3|=3\quad and \displaystyle \quad |-\pi|=\pi\,. |
For positive values of \displaystyle x, the modulus function has no effect, since \displaystyle |x|=x, but for negative \displaystyle x the modulus function changes the sign of \displaystyle x, i.e. \displaystyle |x|=-x (remember that \displaystyle x is negative and therefore \displaystyle -x is positive).
If we draw a graph of \displaystyle y=|x| it will consist of two parts. For \displaystyle x\ge 0 we have \displaystyle y=x, and for \displaystyle x\le 0 we have \displaystyle y=-x\,.
The value of the integral is the area of the region under the graph \displaystyle y=|x| and between \displaystyle x=-1 and \displaystyle x=2.
This region consists of two triangles and we therefore obtain
| \displaystyle \int\limits_{-1}^{2} |x|\,dx = \frac{1}{2}\cdot 1\cdot 1 + \frac{1}{2}\cdot 2\cdot 2 = \frac{5}{2}\,\textrm{.} |