Lösung 1.3:1c

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Lösning 1.3:1c moved to Solution 1.3:1c: Robot: moved page)
Zeile 1: Zeile 1:
-
{{NAVCONTENT_START}}
+
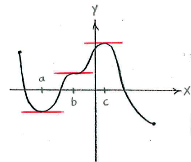
The function has zero derivative at three points,
-
<center> [[Image:1_3_1c-1(3).gif]] </center>
+
<math>x=a</math>,
-
{{NAVCONTENT_STOP}}
+
<math>x=b</math>and
-
{{NAVCONTENT_START}}
+
<math>x=c</math>
-
<center> [[Image:1_3_1c-2(3).gif]] </center>
+
(see picture below), which are therefore the critical points of the function.
-
{{NAVCONTENT_STOP}}
+
-
{{NAVCONTENT_START}}
+
-
<center> [[Image:1_3_1c-3(3).gif]] </center>
+
-
{{NAVCONTENT_STOP}}
+
[[Image:1_3_1_c1.gif|center]]
[[Image:1_3_1_c1.gif|center]]
 +
 +
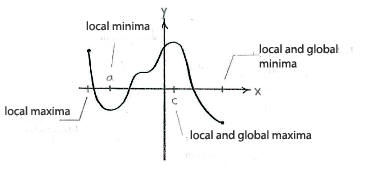
The point
 +
<math>x=b</math>
 +
is an inflexion point because the derivative is positive in a neighbourhood both the left and right.
 +
 +
At the left endpoint of the interval of definition and at
 +
<math>x=c</math>, the function has local maximum points , because the function takes lower values at all points in the vicinity of these points. At the point
 +
<math>x=a</math>
 +
and the right endpoint, the function has local minimum points.
 +
 +
Also, we see that
 +
<math>x=c</math>
 +
is a global maximum (the function takes its largest value there) and the right endpoint is a global minimum.
 +
 +
[[Image:1_3_1_c2.gif|center]]
[[Image:1_3_1_c2.gif|center]]
 +
 +
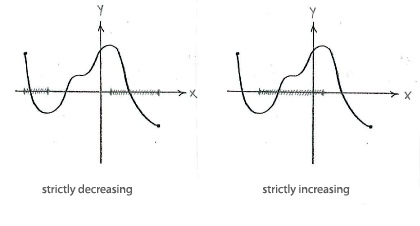
Between the left endpoint and
 +
<math>x=a</math>, as well as between
 +
<math>x=c</math>
 +
and the right endpoint, the function is strictly decreasing (the larger
 +
<math>x</math>
 +
is, the smaller
 +
<math>f\left( x \right)</math>
 +
becomes), whilst the function is strictly increasing between
 +
<math>x=a</math>
 +
and x=c (the graph flattens out at
 +
<math>x=b</math>, but it isn't constant there).
 +
 +
[[Image:1_3_1_c3.gif|center]]
[[Image:1_3_1_c3.gif|center]]

Version vom 09:22, 15. Okt. 2008

The function has zero derivative at three points, \displaystyle x=a, \displaystyle x=band \displaystyle x=c (see picture below), which are therefore the critical points of the function.

The point \displaystyle x=b is an inflexion point because the derivative is positive in a neighbourhood both the left and right.

At the left endpoint of the interval of definition and at \displaystyle x=c, the function has local maximum points , because the function takes lower values at all points in the vicinity of these points. At the point \displaystyle x=a and the right endpoint, the function has local minimum points.

Also, we see that \displaystyle x=c is a global maximum (the function takes its largest value there) and the right endpoint is a global minimum.


Between the left endpoint and \displaystyle x=a, as well as between \displaystyle x=c and the right endpoint, the function is strictly decreasing (the larger \displaystyle x is, the smaller \displaystyle f\left( x \right) becomes), whilst the function is strictly increasing between \displaystyle x=a and x=c (the graph flattens out at \displaystyle x=b, but it isn't constant there).


Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_1.3:1c“