Lösung 3.2:1c
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K (Lösning 3.2:1c moved to Solution 3.2:1c: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
{{NAVCONTENT_START}} | {{NAVCONTENT_START}} | ||
| - | + | By calculation, we obtain | |
| - | + | ||
| + | <math>\begin{align}2z+w &= 2(2+i)+(2+3i)=2\cdot 2 + 2 + (2+3)i\\ | ||
| + | &=6+5i\end{align}</math> | ||
| + | |||
| + | and we can mark this point on the complex plane. | ||
| + | |||
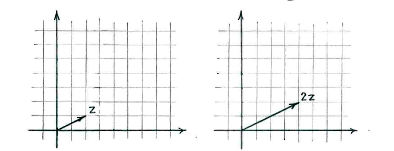
| + | If we treat <math>z</math> and <math>w</math> as vectors, then <math>2z</math> is the vector which has the same direction as <math>z</math>, but is twice as long. | ||
[[Image:3_2_1_c1.gif|center]] | [[Image:3_2_1_c1.gif|center]] | ||
| + | |||
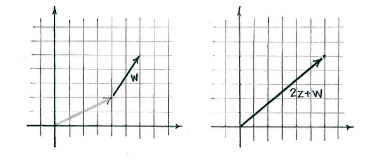
| + | We add <math>w</math> to this vector and get <math>2z+w</math>. | ||
[[Image:3_2_1_c2.gif|center]] | [[Image:3_2_1_c2.gif|center]] | ||
| + | {{NAVCONTENT_STOP}} | ||
Version vom 10:34, 3. Okt. 2008
By calculation, we obtain
\displaystyle \begin{align}2z+w &= 2(2+i)+(2+3i)=2\cdot 2 + 2 + (2+3)i\\ &=6+5i\end{align}
and we can mark this point on the complex plane.
If we treat \displaystyle z and \displaystyle w as vectors, then \displaystyle 2z is the vector which has the same direction as \displaystyle z, but is twice as long.
We add \displaystyle w to this vector and get \displaystyle 2z+w.