Lösung 4.4:7c
Aus Online Mathematik Brückenkurs 1
If we want to solve the equation \displaystyle \cos 3x = \sin 4x, we need an additional result which tells us for which values of u and v the equality \displaystyle \cos u = \sin v holds, but to get that we have to start with the equality \displaystyle \cos u=\cos v.
So, we start by looking at the equality
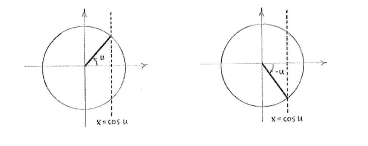
We know that for fixed u there are two angles \displaystyle v=u and \displaystyle v=-u in the unit circle which have the cosine value \displaystyle \cos u, i.e. their x-coordinate is equal to \displaystyle \cos u\,.
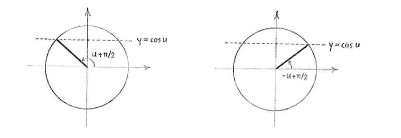
Imagine now that the whole unit circle is rotated anti-clockwise an angle \displaystyle \pi/2. The line \displaystyle x=\cos u will become the line \displaystyle y=\cos u and the angles u and -u are rotated to \displaystyle u+\pi/2 and \displaystyle -u+\pi/2, respectively.
The angles \displaystyle u+\pi/2 and \displaystyle -u+\pi/2 therefore have their y-coordinate, and hence sine value, equal to \displaystyle \cos u. In other words, the equality
holds for fixed u in the unit circle when \displaystyle v = \pm u + \pi/2, and more generally when
For our equation \displaystyle \cos 3x = \sin 4x, this result means that x must satisfy
This means that the solutions to the equation are
where n is an arbitrary integer.